| [1] |
梅凤翔. 约束力学系统的对称性与守恒量[M]. 北京: 北京理工大学出版社, 2004.MEI Fengxiang. Symmetries and Conserved Quantities of Constrained Mechanical Systems[M]. Beijing: Beijing University of Technology Press, 2004.(in Chinese)
|
| [2] |
RICHARD J P. Time-delay systems: an overview of some recent advances and open problems[J]. Automatica, 2003, 39(10): 1667-1694. doi: 10.1016/S0005-1098(03)00167-5
|
| [3] |
胡海岩, 王在华. 非线性时滞动力学系统的研究进展[J]. 力学进展, 1999, 29(4): 501-512. (HU Haiyan, WANG Zaihua. Review nonlinear dynamic systems involving time delays[J]. Advances in Mechanics, 1999, 29(4): 501-512.(in Chinese)
|
| [4] |
马丽, 马瑞楠. 一类随机泛函微分方程带随机步长的EM逼近的渐近稳定[J]. 应用数学和力学, 2019, 40(1): 97-107. (MA Li, MA Ruinan. Almost sure asymptotic stability of the Euler-Maruyama method with random variable stepsizes for stochastic functional differential equations[J]. Applied Mathematics and Mechanics, 2019, 40(1): 97-107.(in Chinese) doi: 10.1007/s10483-019-2403-6
|
| [5] |
ROSENBLUETH J F. Systems with time delay in the calculus of variations: a variational approach[J]. IMA Journal of Mathematical Control and Information, 1988, 5(2): 125-145. doi: 10.1093/imamci/5.2.125
|
| [6] |
CHAN W L, YUNG S P. Sufficient conditions for variational problems with delayed argument[J]. Journal of Optimization Theory and Applications, 1993, 76(1): 131-144. doi: 10.1007/BF00952825
|
| [7] |
FREDERICO G S F, TOMES D F M. Noether’s symmetry theorem for variational and optimal control problems with time delay[J]. Numerical Algebra, 2012, 2(3): 619-630.
|
| [8] |
ZHANG Y, JIN S X. Noether symmetries of dynamics for non-conservative systems with time delay[J]. Acta Physica Sinica, 2013, 62(23): 234502. doi: 10.7498/aps.62.234502
|
| [9] |
JIN S X, ZHANG Y. Noether symmetry and conserved quantity for a Hamilton system with time delay[J]. Chinese Physics B, 2014, 23(5): 339-346.
|
| [10] |
金世欣, 张毅. 相空间中含时滞的非保守力学系统的Noether定理[J]. 中山大学学报(自然科学版), 2014, 53(4): 57-62. (JIN Shixin, ZHANG Yi. Noether theorem for nonconservative mechanical system with time delay in phase space[J]. Acta Scientiarum Naturalium Universitatis Sunyatseni, 2014, 53(4): 57-62.(in Chinese)
|
| [11] |
祖启航, 朱建青, 张毅. 基于微分变分原理的相空间中含时滞的非保守力学系统的守恒律[J]. 广西师范学院学报(自然科学版), 2015, 32(4): 40-46. (ZU Qihang, ZHU Jianqing, ZHANG Yi. A study of conservative laws of nonconservative mechanical system with time delay in phase space based on differential variational principle[J]. Journal of Guangxi Teachers Education University (Natural Science Edition), 2015, 32(4): 40-46.(in Chinese)
|
| [12] |
贺东海. 含时滞的约束力学系统的对称性与守恒量研究[D]. 硕士学位论文. 杭州: 浙江理工大学, 2014.HE Donghai. Symmetries and conserved quantities of constrained mechanical systems with time delay[D]. Master Thesis. Hangzhou: Zhejiang Sci-Tech University, 2014.(in Chinese)
|
| [13] |
LIN F B, MELESHKO S V, FLOOD A E. Symmetries of population balance equations for aggregation, and growth processes[J]. Applied Mathematics and Computation, 2017, 307: 193-203. doi: 10.1016/j.amc.2017.02.048
|
| [14] |
周景润, 傅景礼. 约束Hamilton系统的Lie对称性及其在场论中的应用[J]. 应用数学和力学, 2019, 40(7): 810-822. (ZHOU Jingrun, FU Jingli. Lie symmetry of constrained Hamiltonian systems and its application in field theory[J]. Applied Mathematics and Mechanics, 2019, 40(7): 810-822.(in Chinese)
|
| [15] |
ANRIOPOULOS K, LEACH P G L. Symmetry and singularity properties of second-order ordinary differential equations of Lie’s type Ⅲ[J]. Journal of Mathematical Analysis and Applications, 2007, 328(2): 860-875. doi: 10.1016/j.jmaa.2006.06.006
|
| [16] |
梅凤翔. 分析力学(下卷)[M]. 北京: 北京理工大学出版社, 2013: 32-45.MEI Fengxiang. Analytical Mechanics (Volume Ⅱ)[M]. Beijing: Beijing University of Technology Press, 2013: 32-45.(in Chinese)
|
| [17] |
方建会. 二阶非完整力学系统的Lie对称性与守恒量[J]. 应用数学和力学, 2002, 23(9): 982-986. (FANG Jianhui. Lie symmetries and conserved quantities of second-order nonholonomic mechanical system[J]. Applied Mathematics and Mechanics, 2002, 23(9): 982-986.(in Chinese) doi: 10.3321/j.issn:1000-0887.2002.09.014
|




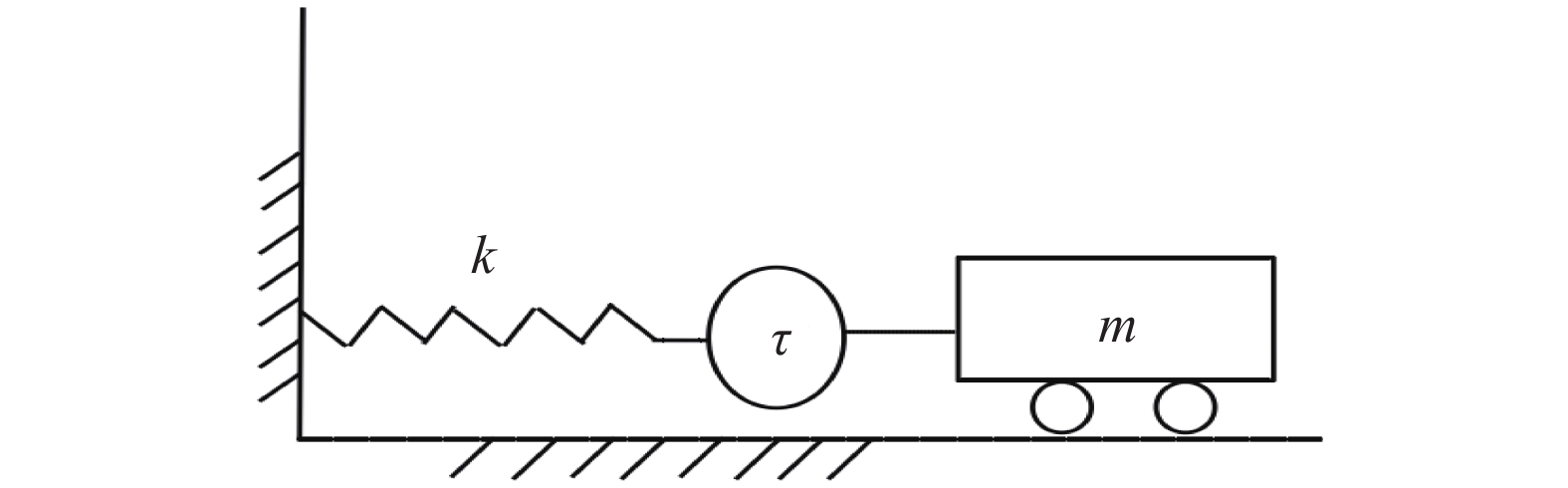
 下载:
下载:

 渝公网安备50010802005915号
渝公网安备50010802005915号