Transient Primary Resonance Phase-Frequency Characteristics of Stay Cables With Different Tensions
-
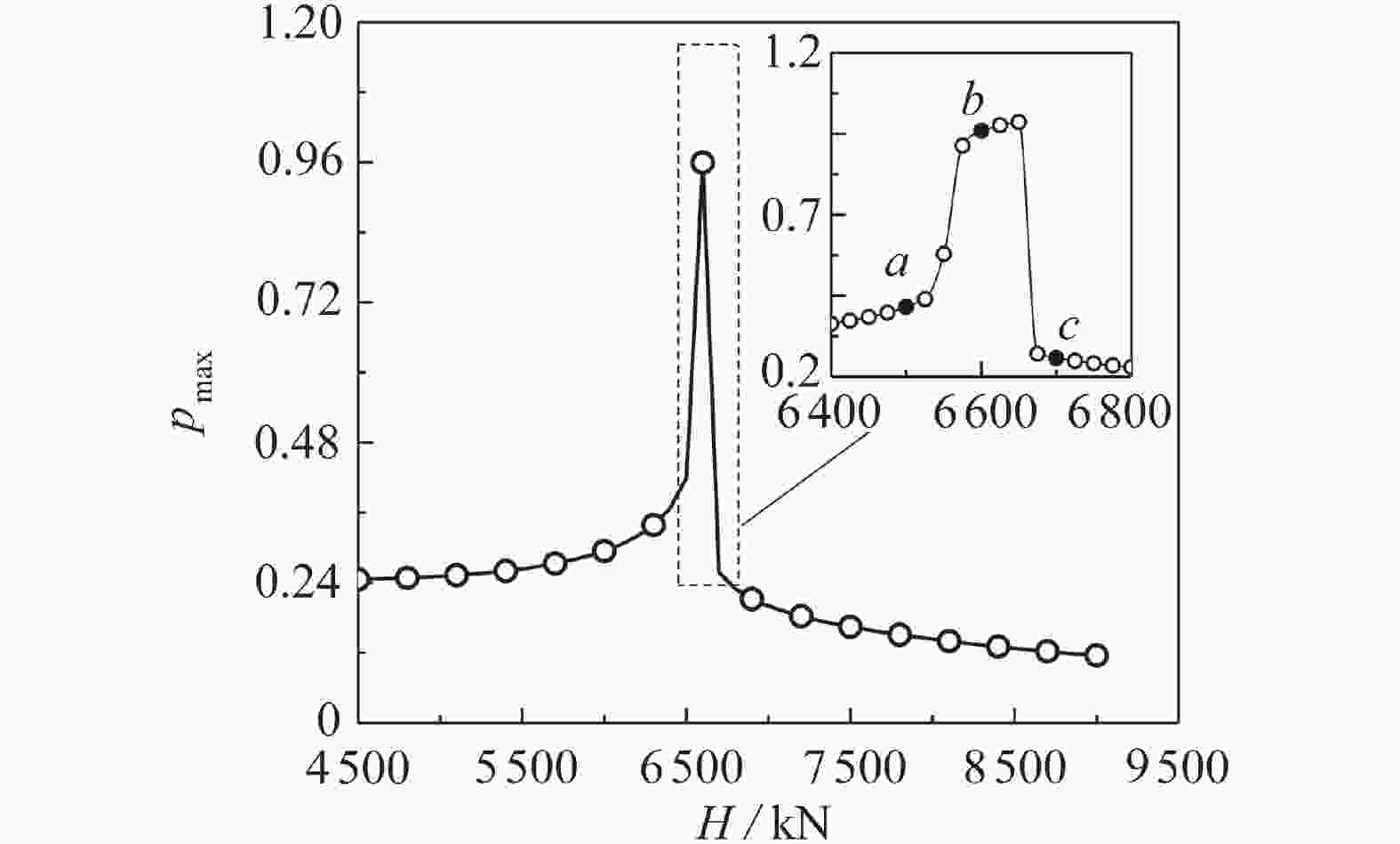
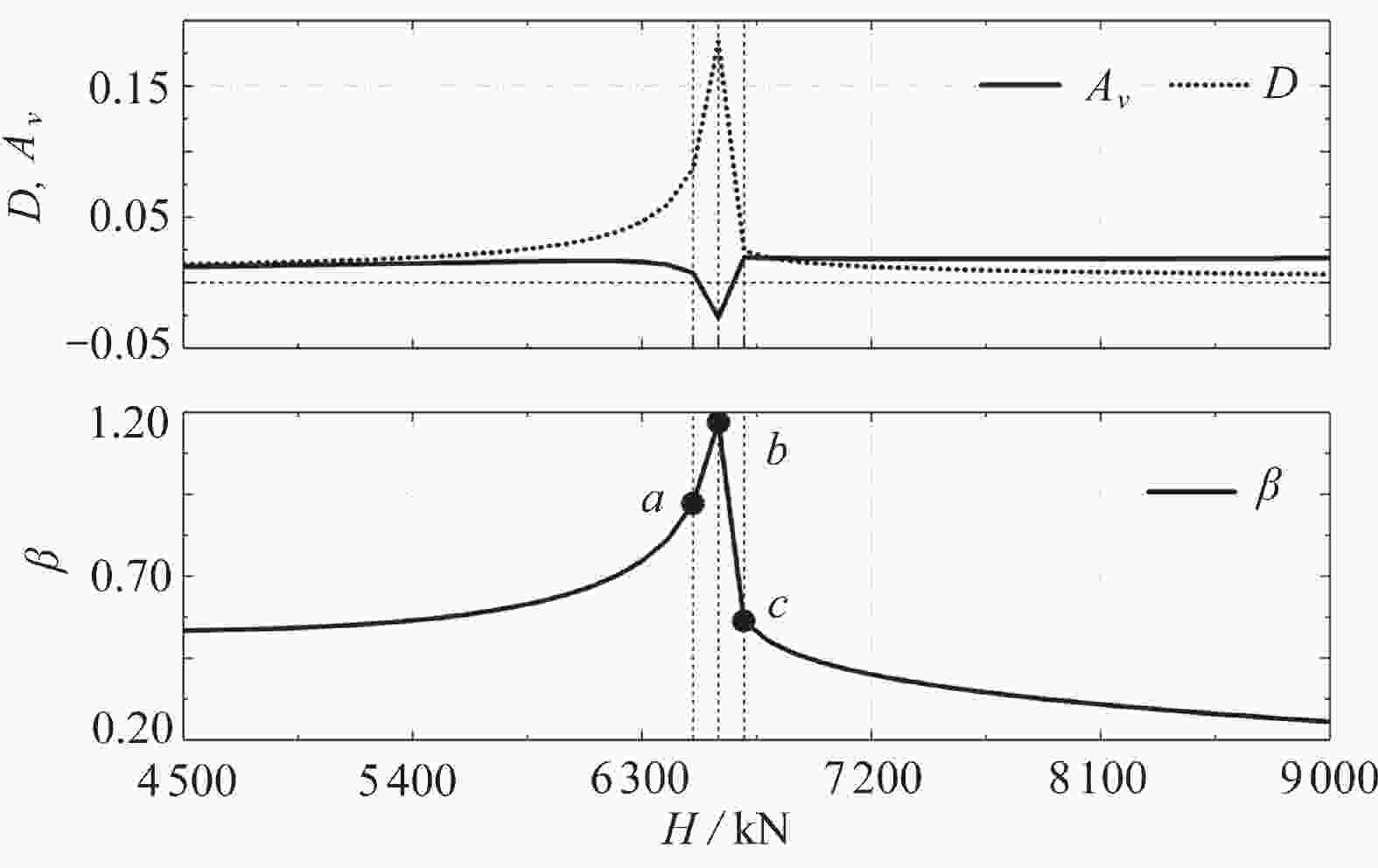
摘要: 考虑拉索垂度及几何非线性，研究了不同索力拉索的瞬时相频特性。利用斜拉索面内分布激励下的运动控制方程，采用多尺度法对微分方程进行摄动求解，分别得到面内、外主共振响应的近似解析式，再采用Hilbert变换得到响应与激励的瞬时相位差及其幅值。研究了不同索力下，响应与激励的瞬时相位差的变化规律及其原因。研究表明：面外主共振响应与激励间保持恒定的相位差，而面内响应与激励的瞬时相位差与索弹性参数和垂度等有关，微小的索力变化可能导致瞬时相频特性的明显改变。主要原因是面内响应的近似解中存在两倍频项和漂移项，前者使响应瞬时相位在单个周期内出现两次正负交替，后者决定面内响应与激励瞬时相位差的最大值及其变化规律。Abstract: The transient phase-frequency characteristics of stay cables with different cable forces were studied in view of the cable sag and geometric nonlinearity. The method of multiple scales was used to solve the ordinary differential equations of motion for cables subjected to in-plane distributed excitations, and the approximate analytical expressions of in-plane and out-of-plane primary resonance responses were obtained respectively. Then, the transient phase difference and its amplitude between the response and the excitation were obtained through the Hilbert transform. The rule and reason for the transient phase difference between the response and the excitation under different cable forces were studied. The results show that, the phase difference between the out-of-plane response and the excitation is constant, while for the in-plane one it is related to the elastic parameters and the sag of the cable. A small change in cable tension may result in a significant change in the transient phase-frequency characteristics. The main reason is that there are a twice-frequency term and a drift term in the approximate solution of the in-plane response, the former makes the transient phase of response appear twice positive-negative alternations in a single cycle, and the latter determines the maximum value and the variation law of the transient phase difference between the in-plane response and excitation.
-
图 1 分布外激励下斜拉索振动简化模型
Figure 1. Simplified model of vibration of cable under distributed external excitation
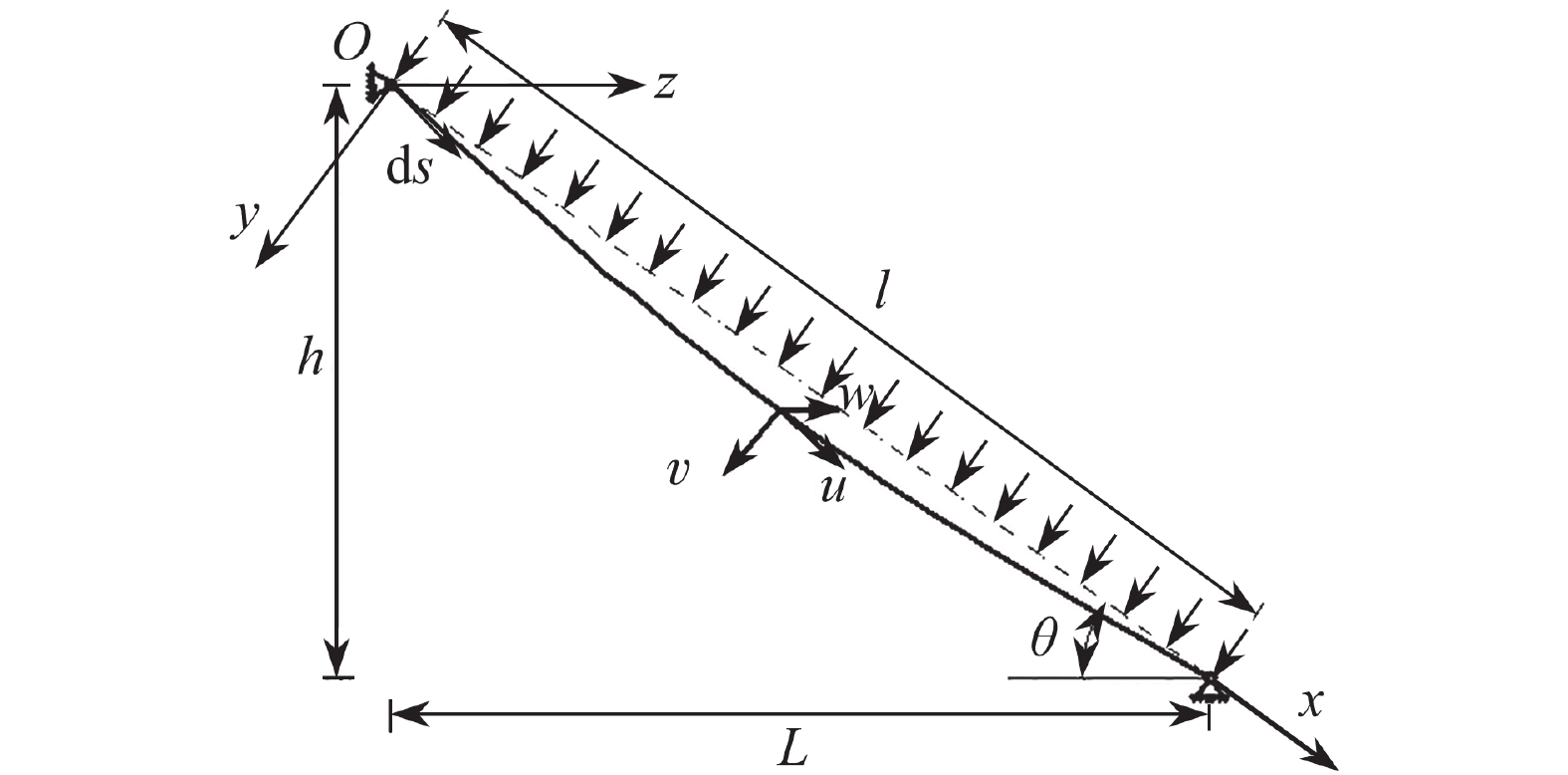
表 1 Normandie桥索参数
Table 1. Cable parameters of the Normandie bridge
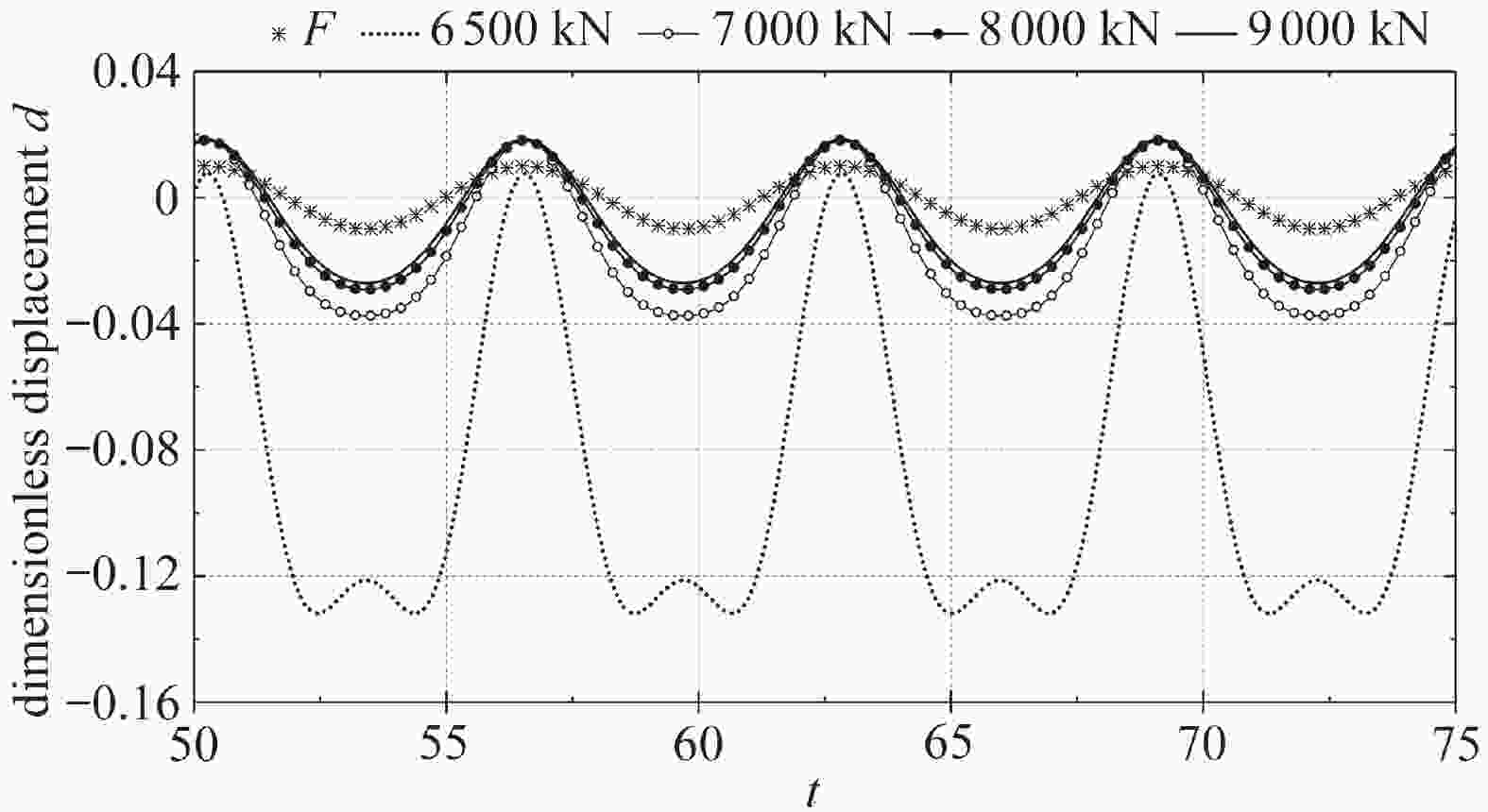
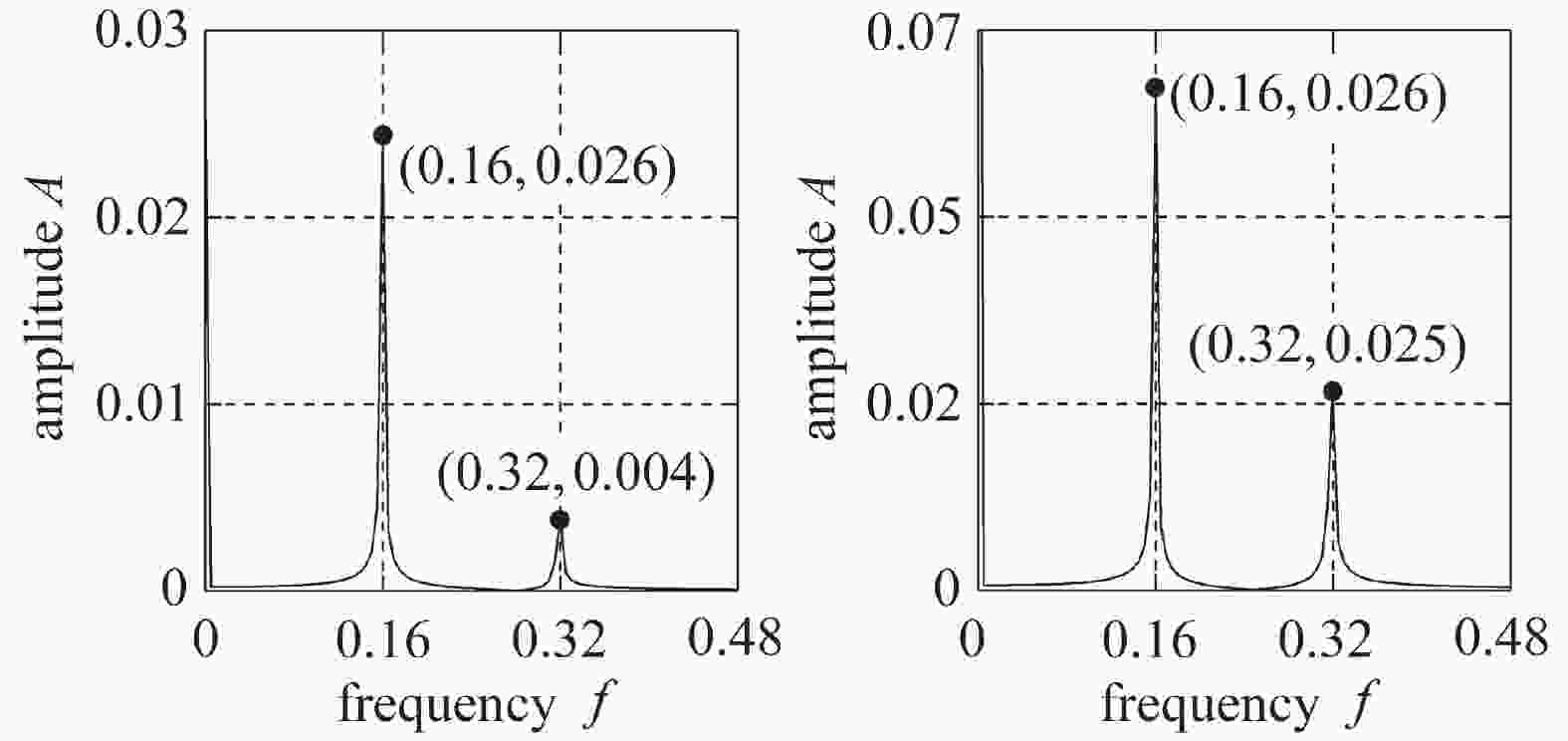
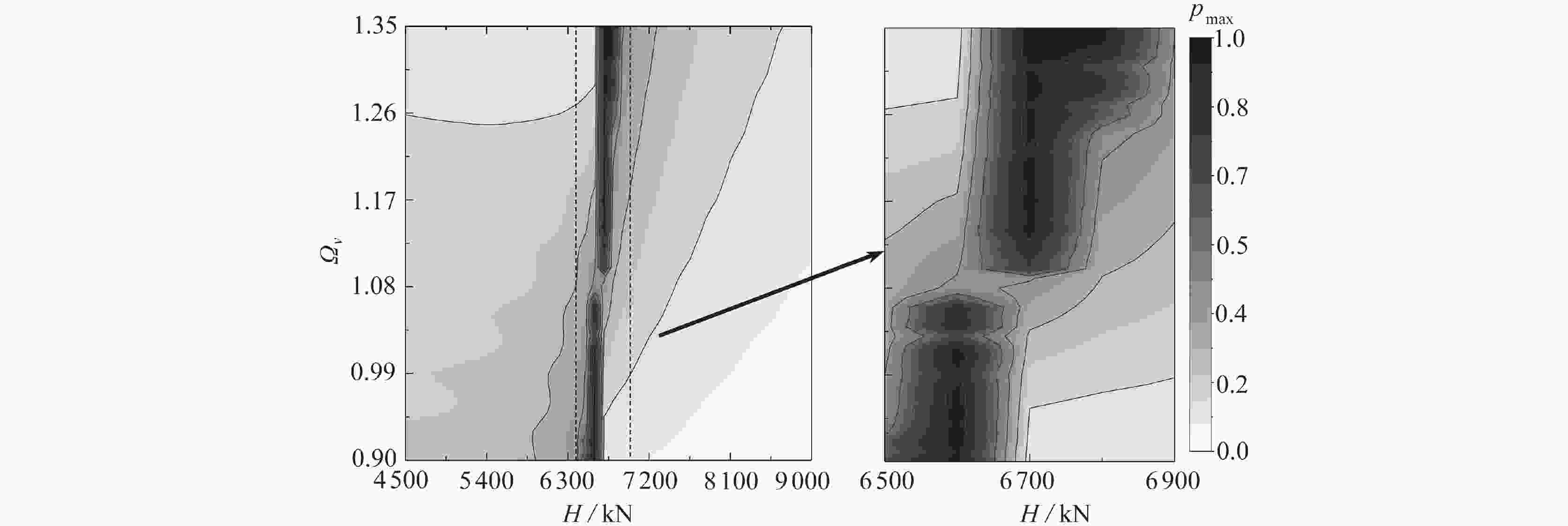
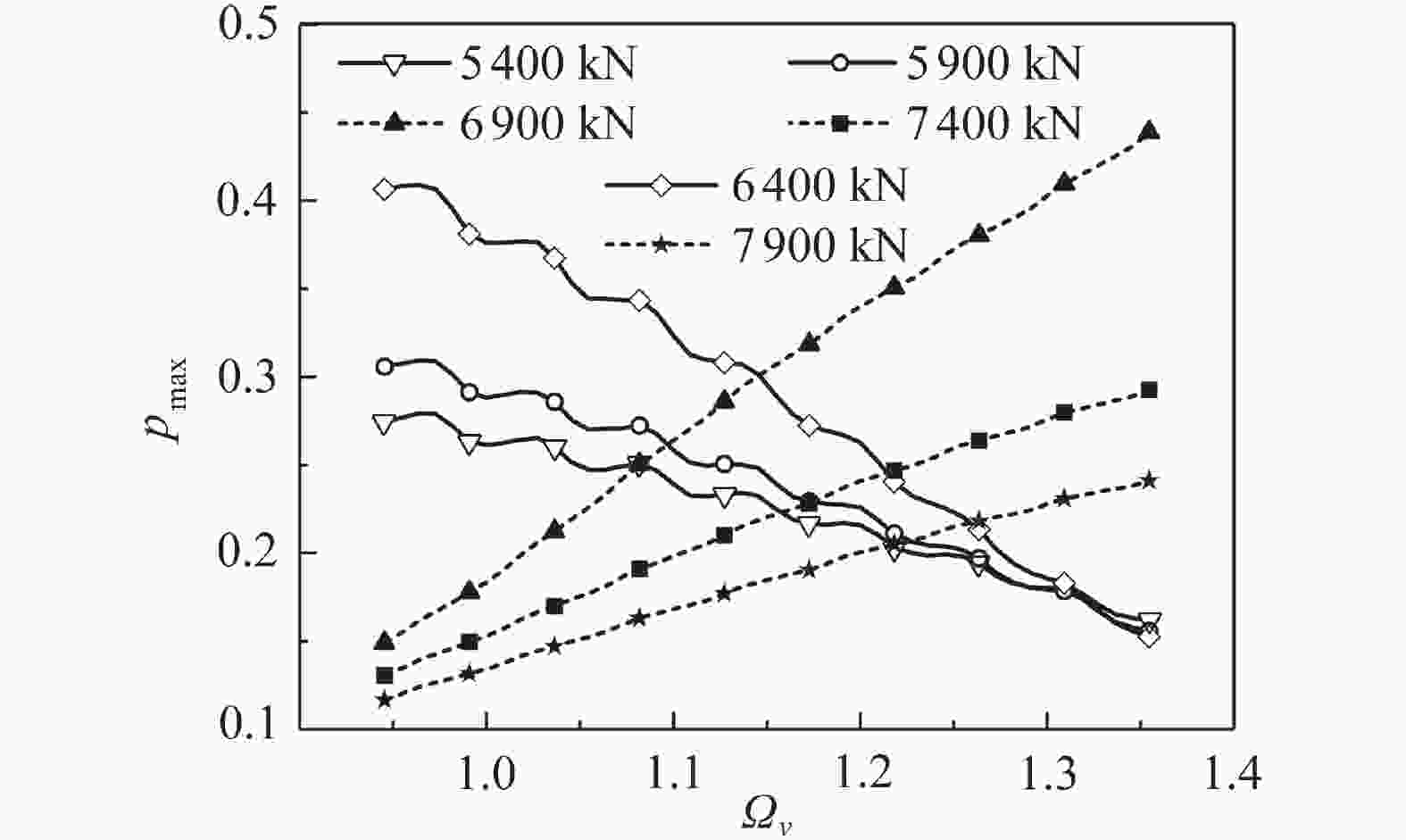
l/m m/(kg·m−1) H/kN A/m2 E/Pa θ/(°) C 440 136 8 000 1.53×10−2 1.9×1011 17.5 0.001 
下载: 导出CSV
-
[1] IRVINE H M. Cable Structures[M]. Cambridge: the MIT Press, 1981: 1-152. [2] 康厚军, 郭铁丁, 赵跃宇. 大跨度斜拉桥非线性振动模型与理论研究进展[J]. 力学学报, 2016, 48 (3): 519-535. (KANG Houjun, GUO Tieding, ZHAO Yueyu. Review on nonlinear vibration and modeling of large span cable-stayed bridge[J]. Chinese Journal of Theoretical and Applied Mechanics, 2016, 48 (3): 519-535.(in Chinese) doi: 10.6052/0459-1879-15-436 [3] 付英. 基础激励下桥梁斜拉索的非线性振动[J]. 动力学与控制学报, 2010, 8 (1): 57-61. (FU Ying. Nonlinear vibration of cables in cable-stayed bridge under foundation excitation[J]. Journal of Dynamics and Control, 2010, 8 (1): 57-61.(in Chinese) doi: 10.3969/j.issn.1672-6553.2010.01.012 [4] 吴娟, 钱有华. 一类弦-梁耦合非线性振动系统的动力学数值模拟研究[J]. 动力学与控制学报, 2018, 16 (5): 403-410. (WU Juan, QIAN Youhua. Numerical simulation research on dynamics of a string-beam coupled nonlinear vibration system[J]. Journal of Dynamics and Control, 2018, 16 (5): 403-410.(in Chinese) doi: 10.6052/1672-6553-2018-020 [5] 丛云跃, 康厚军, 郭铁丁, 等. CFRP索斜拉桥面内自由振动的多索梁模型及模态分析[J]. 动力学与控制学报, 2017, 15 (6): 494-504. (CONG Yunyue, KANG Houjun, GUO Tieding, et al. A multiple cable-beam model and modal analysis on in-plane free vibration of cable-stayed bridge with CFRP cables[J]. Journal of Dynamics and Control, 2017, 15 (6): 494-504.(in Chinese) [6] NI Y Q, WANG X Y, CHEN Z Q, et al. Field observations of rain-wind-induced cable vibration in cable-stayed Dongting Lake Bridge[J]. Journal of Wind Engineering and Industrial Aerodynamics, 2007, 95(5): 303-328. doi: 10.1016/j.jweia.2006.07.001 [7] MATSUMOTO M, SHIRAISHI N, SHIRATO H. Rain-wind induced vibration of cables of cable-stayed bridges[J]. Journal of Wind Engineering and Industrial Aerodynamics, 1992, 43(1/3): 2011-2022. doi: 10.1016/0167-6105(92)90628-N [8] SAVOR Z, RADIC J, HRELJA G. Cable vibrations at Dubrovnik bridge[J]. Bridge Structures, 2006, 2(2): 97-106. doi: 10.1080/15732480600855800 [9] 符旭晨, 周岱, 吴筑海. 斜拉索的风振与减振[J]. 振动与冲击, 2004, 23 (3): 29-32. (FU Xuchen, ZHOU Dai, WU Zhuhai. Study on wind induced vibration and vibration reduction of stayed cables[J]. Journal of Vibration and Shock, 2004, 23 (3): 29-32.(in Chinese) doi: 10.3969/j.issn.1000-3835.2004.03.008 [10] REGA G, ALAGGIO R, BENEDETTINI F. Experimental investigation of the nonlinear response of a hanging cable, part P: local analysis[J]. Nonlinear Dynamics, 1997, 14(2): 89-117. doi: 10.1023/A:1008246504104 [11] ZHAO Y, HUANG C, CHEN L, et al. Nonlinear vibration behaviors of suspended cables under two-frequency excitation with temperature effects[J]. Journal of Sound and Vibration. 2018, 416: 279-294. [12] WANG L H, ZHAO Y Y. Nonlinear interactions and chaotic dynamics of suspended cables with three-to-one internal resonances[J]. International Journal of Solids and Structures, 2006, 43(25): 7800-7819. [13] BOSSENS F, PREUMONT A. Active tendon control of cable-stayed bridges: a large-scale demonstration[J]. Earthquake Engineering & Structural Dynamics, 2001, 30(7): 961-979. [14] 孙测世. 大跨度斜拉桥非线性振动试验研究[D]. 博士学位论文. 长沙: 湖南大学, 2015.SUN Ceshi. Experimental study of nonlinear vibrations of long-span cable-stayed bridge[D]. PhD Thesis. Changsha: Hunan University, 2015. （in Chinese） [15] 赵珧冰, 林恒辉, 黄超辉, 等. 温度场中悬索受多频激励组合联合共振响应研究[J]. 振动与冲击, 2019, 38 (3): 215-221. (ZHAO Yaobing, LIN Henghui, HUANG Chaohui, et al. Combined joint resonance responses of suspended cable subject to multi-frequency excitation in thermal environment[J]. Journal of Vibration and Shock, 2019, 38 (3): 215-221.(in Chinese) [16] PERLIKOWSKI P, KAPITANIAK M, CZOLCZYNSKI K, et al. Chaos in coupled clocks[J]. International Journal of Bifurcation and Chaos, 2012, 22(12): 1250288. doi: 10.1142/S0218127412502884 [17] WU Y, WANG N, LI L, et al. Anti-phase synchronization of two coupled mechanical metronomes[J]. Chaos: an Interdisciplinary Journal of Nonlinear Science, 2012, 22(2): 023146. doi: 10.1063/1.4729456 [18] CZOLCZYNSKI K, PERLIKOWSKI P, STEFANSKI A, et al. Synchronization of the self-excited pendula suspended on the vertically displacing beam[J]. Communications in Nonlinear Science and Numerical Simulation, 2013, 18(2): 386-400. doi: 10.1016/j.cnsns.2012.07.007 [19] KAPITANIAK M, PERLIKOWSKI P, KAPITANIAK T. Synchronous motion of two vertically excited planar elastic pendula[J]. Communications in Nonlinear Science and Numerical Simulation, 2013, 18(8): 2088-2096. doi: 10.1016/j.cnsns.2012.12.030 [20] WANG L, ZHAO Y. Large amplitude motion mechanism and non-planar vibration character of stay cables subject to the support motions[J]. Journal of Sound and Vibration, 2009, 327(1/2): 121-133. [21] SUN C, ZHAO Y, WANG Z, et al. Effects of longitudinal girder vibration on non-linear cable responses in cable-stayed bridges[J]. European Journal of Environmental and Civil Engineering, 2015, 21(1): 1-14. [22] WARNITCHAI P, FUJINO Y, SUSUMPOW T. A non-linear dynamic model for cables and its application to a cable-structure system[J]. Journal of Sound and Vibration, 1995, 187(4): 695-712. [23] WU Q, TAKAHASHI K, NAKAMURA S. Formulae for frequencies and modes of in-plane vibrations of small-sag inclined cables[J]. Journal of Sound and Vibration, 2005, 279(3/5): 1155-1169. [24] KANG H J, ZHU H P, ZHAO Y Y, et al. In-plane non-linear dynamics of the stay cables[J]. Nonlinear Dynamics, 2013, 73(3): 1385-1398. doi: 10.1007/s11071-013-0871-2 [25] 陈水生, 孙炳楠, 胡隽. 斜拉索受轴向激励引起的面内参数振动分析[J]. 振动工程学报, 2002, 15(2): 144-150. (CHEN Shuisheng, SUN Bingnan, HU Jun. Analysis of stayed-cable vibration caused by axial excitation[J]. Journal of Vibration Engineering, 2002, 15(2): 144-150.(in Chinese) doi: 10.3969/j.issn.1004-4523.2002.02.005 [26] 徐夷鹏, 任志明, 李振春, 等. 一阶近似瞬时频率时间域声波全波形反演[J]. 石油地球物理勘探, 2020, 55(5): 1029-1038. (XU Yipeng, REN Zhiming, LI Zhenchun, et al. Full waveform inversion of time-domain acoustic wave based on first-order approximate instantaneous frequency[J]. Oil Geophysical Prospecting, 2020, 55(5): 1029-1038.(in Chinese) -
图(10) / 表(1)
计量
- 文章访问数: 654
- HTML全文浏览量: 271
- PDF下载量: 51
- 被引次数: 0
渝公网安备50010802005915号