Buckling Behaviors of Elastomers With Periodic Elliptical Holes Under Negative Pressure Activation
-
摘要:
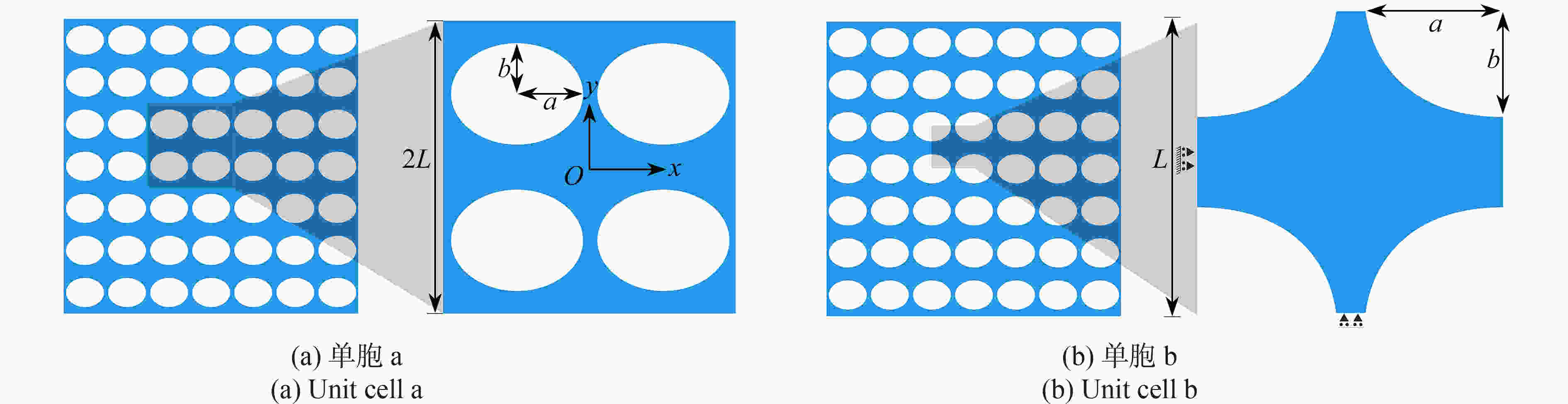
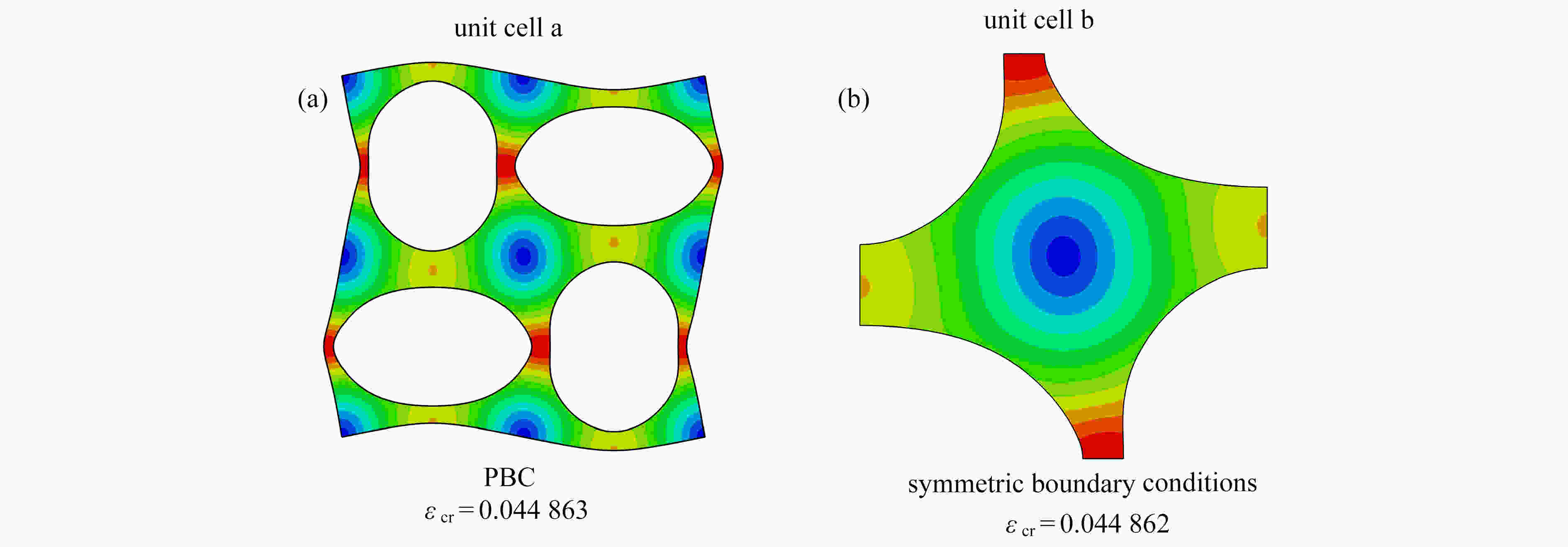
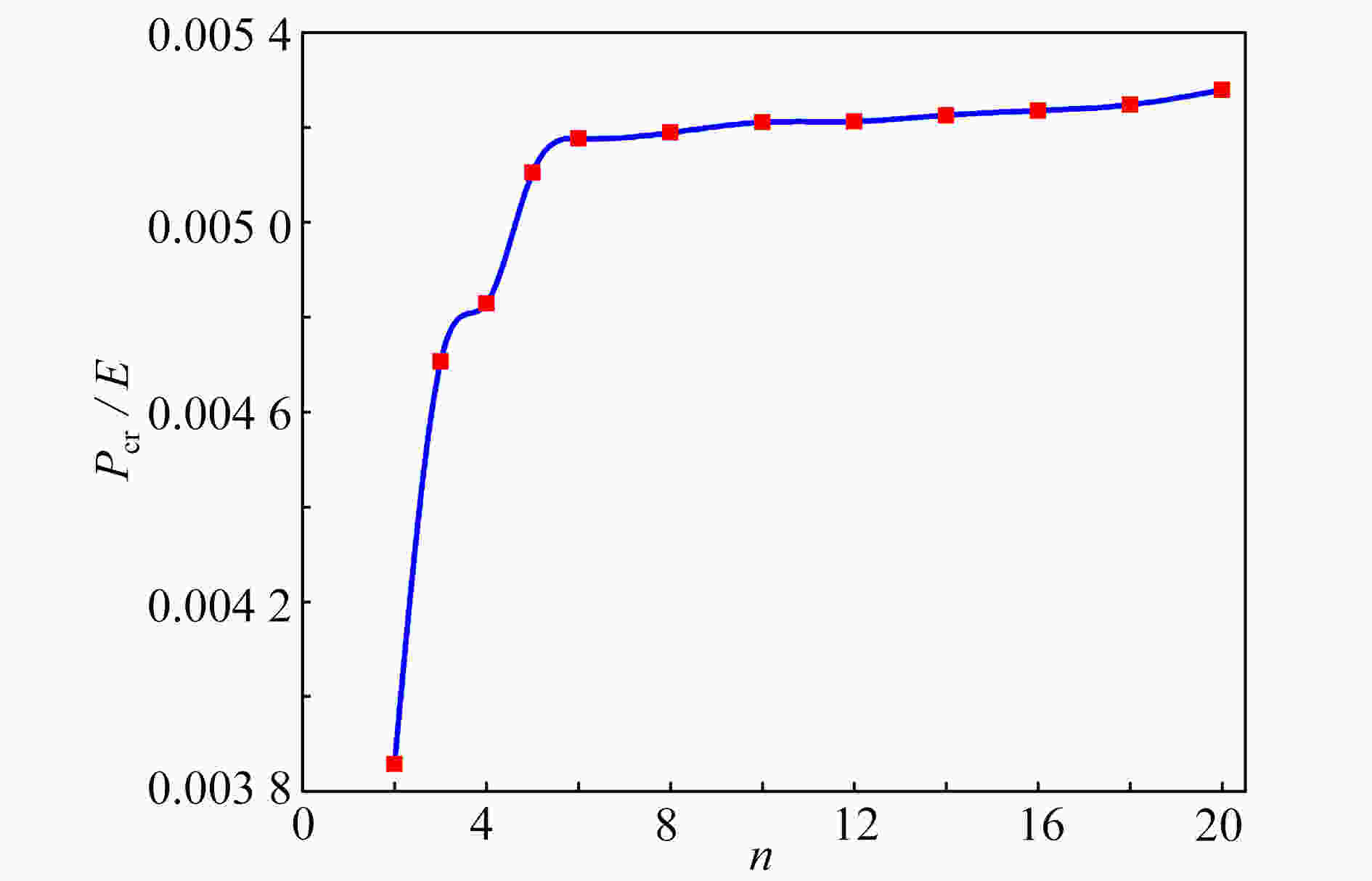
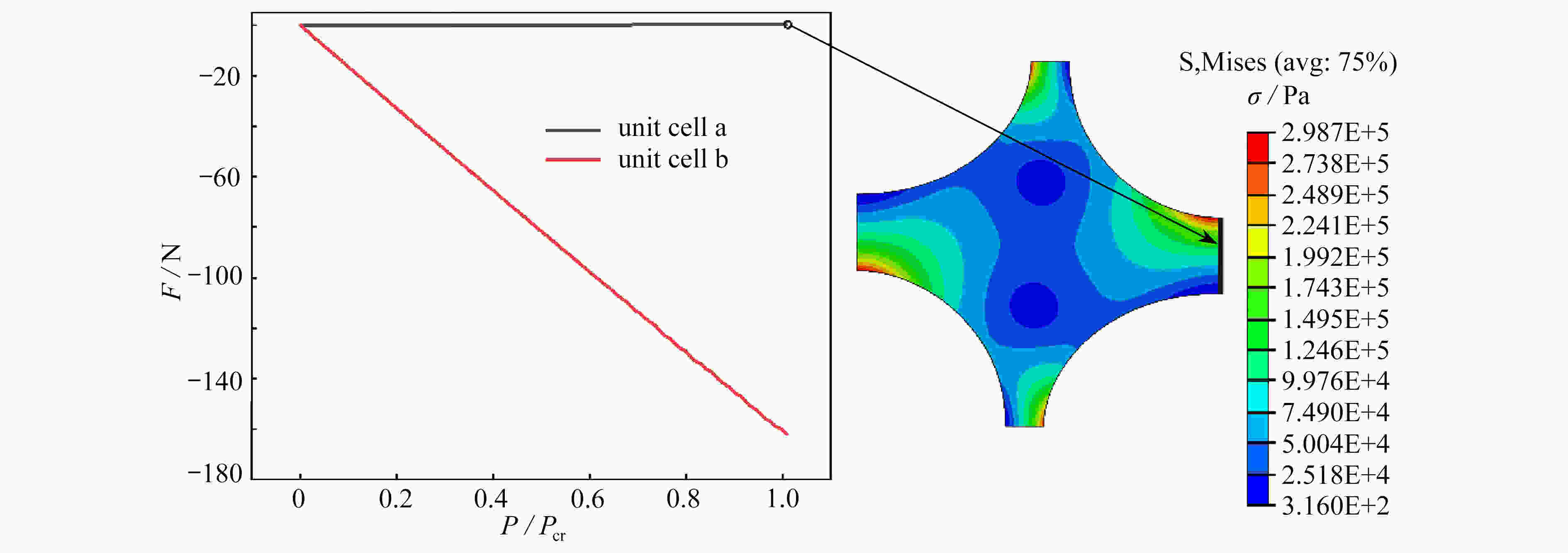
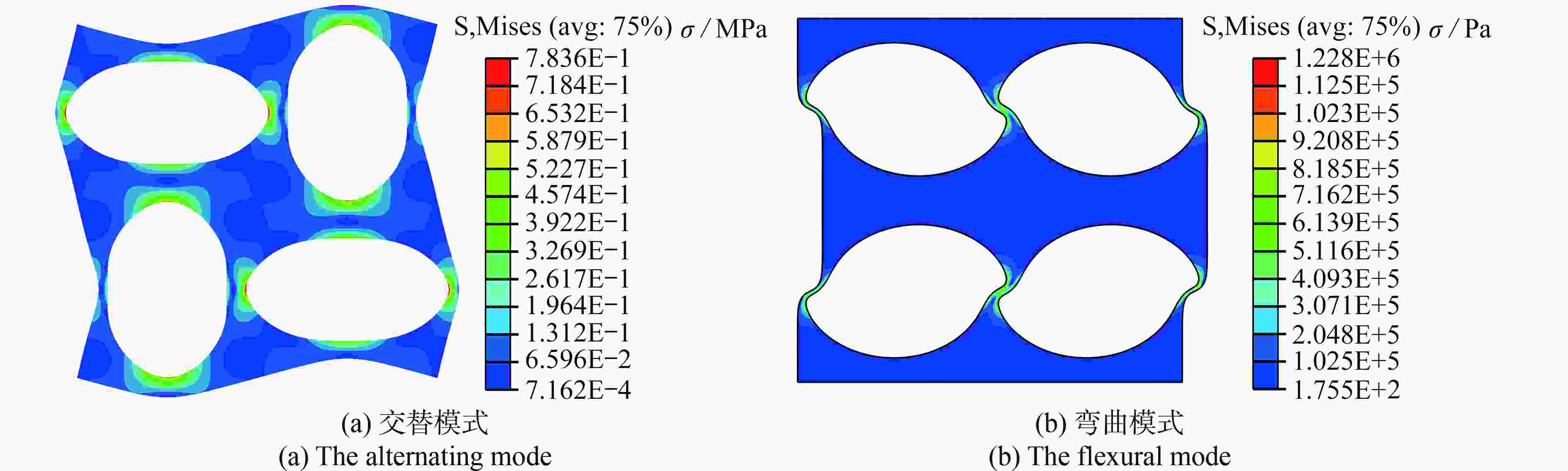
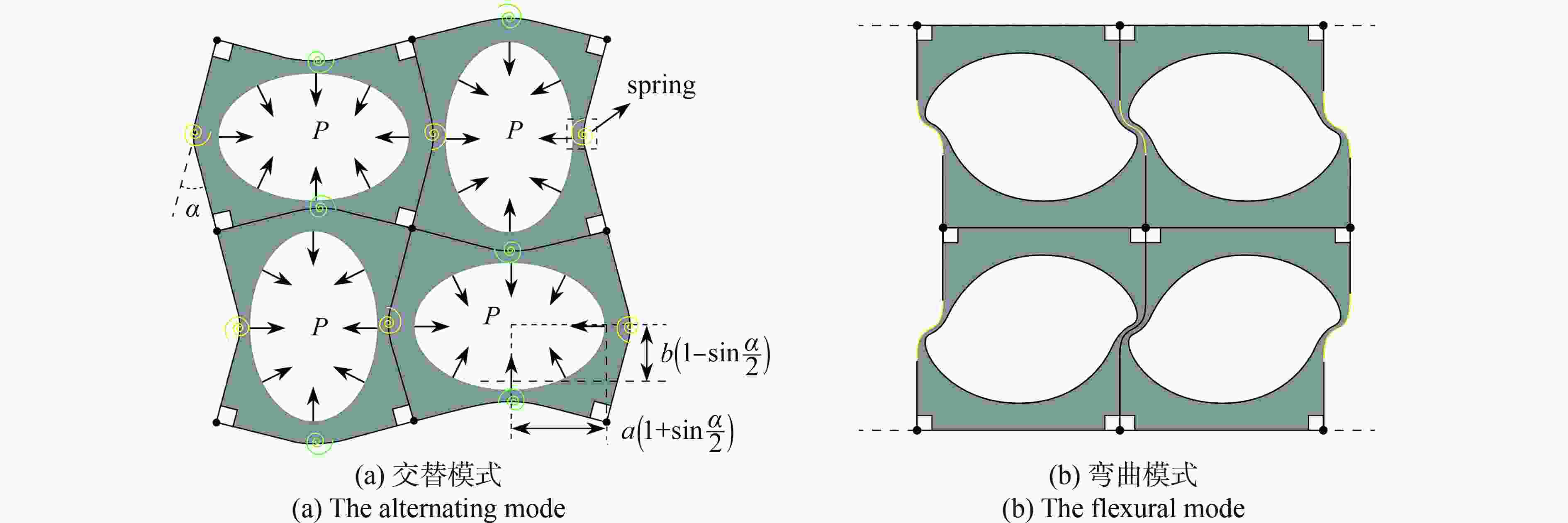
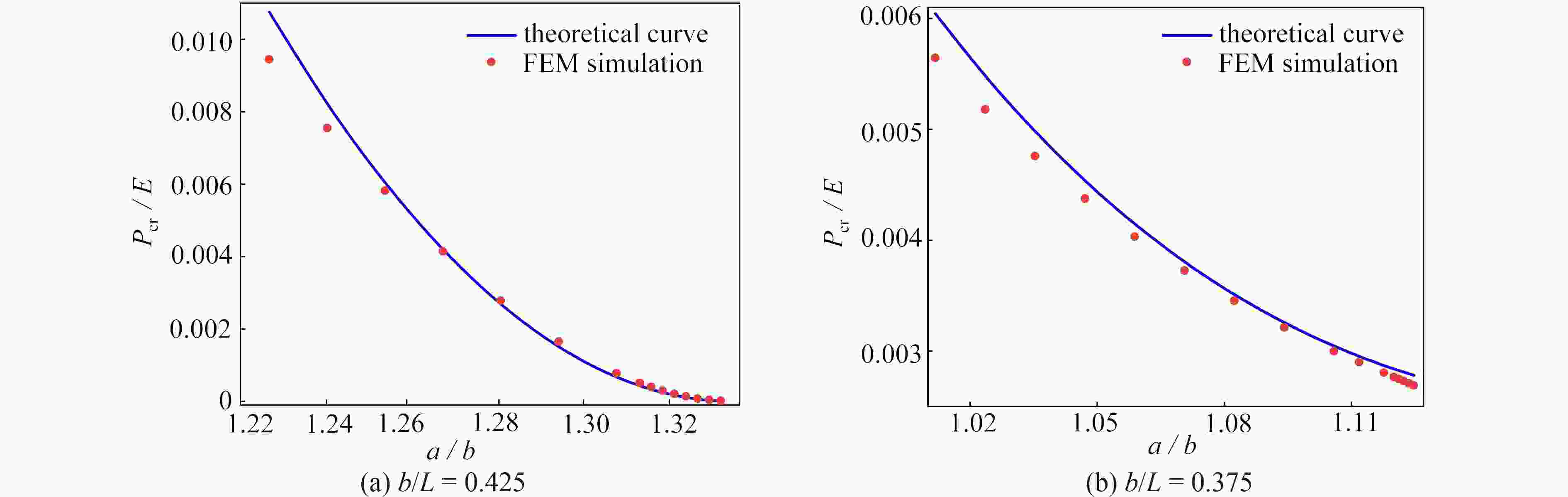
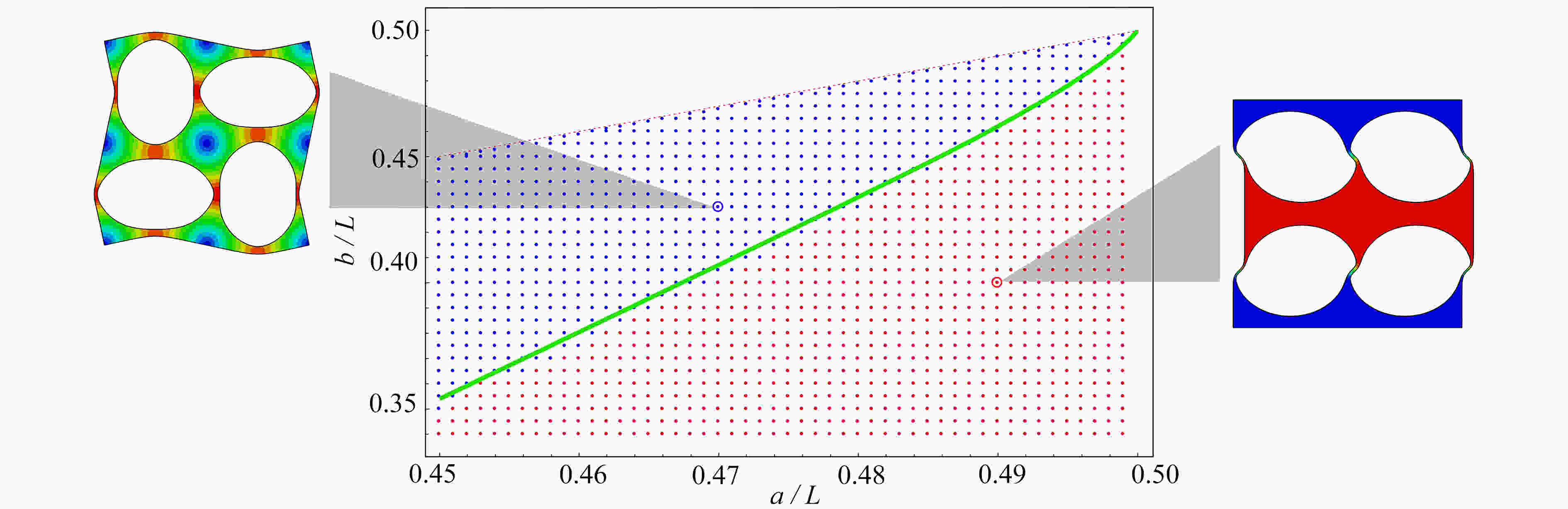
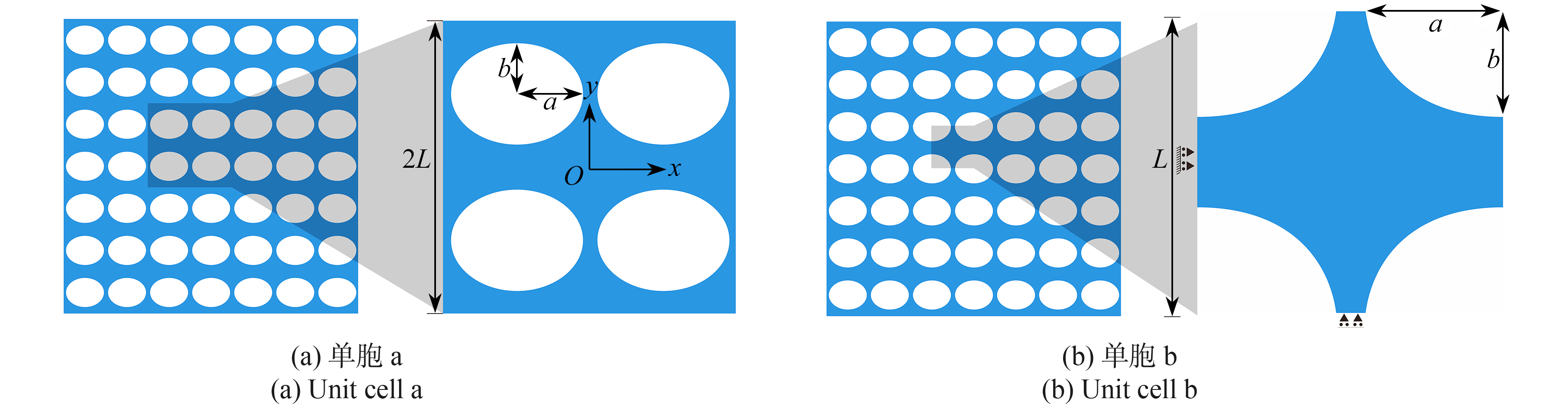
基于数值模拟与理论分析，研究了含周期性椭圆孔二维结构的屈曲行为。针对不同的屈曲模态，建立理论模型进行模态分析。结果表明，改变孔的几何参数，椭圆孔结构的屈曲模态会随之发生转换，理论分析与数值结果吻合良好。此外，在数值模拟中，与位移加载不同，负压激励下的单胞需要考虑力边界条件的修正，以确保其满足完备性条件。已有工作在单胞选择中常存在问题，导致错误结果。针对上述问题研究了不同单胞所对应的边界条件，并结合有限结构进行了分析与讨论。
Abstract:The buckling behaviors of 2D structures with periodic elliptical holes were studied through numerical simulations and theoretical analysis. A theoretical model was established for the modal analysis corresponding to different buckling modes. The analysis results indicate that, there is a transformation between the buckling modes of the 2D structure with elliptical holes, with the change of the geometrical parameters of the holes. The theoretical analysis and the numerical results match up. Furthermore, in the numerical simulation, a modified force boundary condition for a unit cell under negative pressure activation, being different from the displacement loading, should be considered to ensure the completeness conditions are fulfilled. The confusion in the application of appropriate boundary conditions for the unit cell will result in errors. The choice of the unit cell and the derivation of correct boundary conditions, combined with finite structures, were discussed.
-
Key words:
- negative pressure activation /
- porous elastomer /
- metamaterial /
- buckling
-
图 1 二维正方排布椭圆孔周期结构的不同单胞取法
Figure 1. Various methods of selecting unit cells for the periodic structure with elliptical holes in 2D tetragonal lattice arrangement
图 2 单胞a、单胞b与修正后的单胞b在负压加载下的临界压力
Figure 2. Critical pressures of unit cell a， unit cell b and modified unit cell b under the negative pressure
图 3 单胞a与单胞b在单轴位移加载下的临界应变
Figure 3. Critical strains of unit cell a and unit cell b under the loading of uniaxial displacement
图 4 有限结构与单胞a在条带截面的法向合力
Figure 4. Normal resultant forces of the finite structure and unit cell a at the strip section
图 5 有限结构大小对其临界力的影响
Figure 5. The influence of the finite structure size on its critical force
图 6 单胞a与单胞b在条带截面的法向合力
注 为了解释图中的颜色，读者可以参考本文的电子网页版本，后同。
Figure 6. Normal resultant forces of unit cell a and unit cell b at the strip section
图 10 理论临界压力与数值模拟结果的比较
Figure 10. Comparison of theoretical critical pressures and numerical simulation results
-
[1] LEE J H, SINGER J P, THOMAS E L. Micro-/nanostructured mechanical metamaterials[J]. Advanced Materials, 2012, 24(36): 4782-4810. doi: 10.1002/adma.201201644 [2] COULAIS C, TEOMY E, DE REUS K, et al. Combinatorial design of textured mechanical metamaterials[J]. Nature, 2016, 535(7613): 529-532. doi: 10.1038/nature18960 [3] 陈启勇, 胡少伟, 张子明. 基于声子晶体理论的弹性地基梁的振动特性研究[J]. 应用数学和力学, 2014, 35(1): 29-38. (CHEN Qiyong, HU Shaowei, ZHANG Ziming. Research on the vibration property of the beam on elastic foundation based on the PCs theory[J]. Applied Mathematics and Mechanics, 2014, 35(1): 29-38.(in Chinese) doi: 10.3879/j.issn.1000-0887.2014.01.004 [4] PELRINE R, KORNBLUH R, PEI Q B, et al. High-speed electrically actuated elastomers with strain greater than 100%[J]. Science, 2000, 287(5454): 836-839. doi: 10.1126/science.287.5454.836 [5] BROCHU P, PEI Q B. Advances in dielectric elastomers for actuators and artificial muscles[J]. Macromolecular Rapid Communications, 2010, 31(1): 10-36. doi: 10.1002/marc.200900425 [6] BERTOLDI K, BOYCE M C, DESCHANEL S, et al. Mechanics of deformation-triggered pattern transformations and superelastic behavior in periodic elastomeric structures[J]. Journal of the Mechanics and Physics of Solids, 2008, 56(8): 2642-2668. doi: 10.1016/j.jmps.2008.03.006 [7] WANG G, LI M, ZHOU J. Switching of deformation modes in soft mechanical metamaterials[J]. Soft Materials, 2016, 14(3): 180-186. doi: 10.1080/1539445X.2016.1178143 [8] MULLIN T, DESCHANEL S, BERTOLDI K, et al. Pattern transformation triggered by deformation[J]. Physical Review Letters, 2007, 99(8): 084301. doi: 10.1103/PhysRevLett.99.084301 [9] BERTOLDI K, REIS P M, WILLSHAW S, et al. Negative Poisson’s ratio behavior induced by an elastic instability[J]. Advanced Materials, 2010, 22(3): 361-366. doi: 10.1002/adma.200901956 [10] HU J, HE Y, LEI J, et al. Novel mechanical behavior of periodic structure with the pattern transformation[J]. Theoretical and Applied Mechanics Letters, 2013, 3(5): 054007. doi: 10.1063/2.1305407 [11] BERTOLDI K, BOYCE M C. Mechanically triggered transformations of phononic band gaps in periodic elastomeric structures[J]. Physical Review B, 2008, 77(5): 439-446. [12] HU J, HE Y, LEI J, et al. Mechanical behavior of composite gel periodic structures with the pattern transformation[J]. Structural Engineering and Mechanics, 2014, 50(5): 605-616. doi: 10.12989/sem.2014.50.5.605 [13] LI J, SLESARENKO V, RUDYKH S. Auxetic multiphase soft composite material design through instabilities with application for acoustic metamaterials[J]. Soft Matter, 2018, 14(30): 6171-6180. doi: 10.1039/C8SM00874D [14] LI J, WANG Y T, CHEN W Q, et al. Harnessing inclusions to tune post-buckling deformation and bandgaps of soft porous periodic structures[J]. Journal of Sound and Vibration, 2019, 459: 114848. doi: 10.1016/j.jsv.2019.114848 [15] CHEN K L, CAO Y P, ZHANG M G, et al. Indentation-triggered pattern transformation in hyperelastic soft cellular solids[J]. Comptes Rendus Mécanique, 2014, 342(5): 292-298. [16] CHEN Y, SCARPA F, REMILLAT C, et al. Curved Kirigami SILICOMB cellular structures with zero Poisson’s ratio for large deformations and morphing[J]. Journal of Intelligent Material Systems and Structures, 2013, 25(6): 731-743. [17] WANG P, SHIM J, BERTOLDI K. Effects of geometric and material nonlinearities on tunable band gaps and low-frequency directionality of phononic crystals[J]. Physical Review B, 2013, 88(1): 2466-2472. [18] SHIM J, WANG P, BERTOLDI K. Harnessing instability-induced pattern transformation to design tunable phononic crystals[J]. International Journal of Solids and Structures, 2015, 58: 52-61. doi: 10.1016/j.ijsolstr.2014.12.018 [19] JOHNSON C G, JAIN U, HAZEL A L, et al. On the buckling of an elastic holey column[J]. Proceedings of the Royal Society A: Mathematical Physical and Engineering Sciences, 2017, 473(2207): 20170477. doi: 10.1098/rspa.2017.0477 [20] CHEN Y C, JIN L H. Geometric role in designing pneumatically actuated pattern-transforming metamaterials[J]. Extreme Mechanics Letters, 2018, 23: 55-66. doi: 10.1016/j.eml.2018.08.001 -
下载:
计量
- 文章访问数: 878
- HTML全文浏览量: 320
- PDF下载量: 86
- 被引次数: 0
渝公网安备50010802005915号