A Novel Localized Meshless Collocation Method for Numerical Simulation of Flume Dynamic Characteristics
-
摘要:
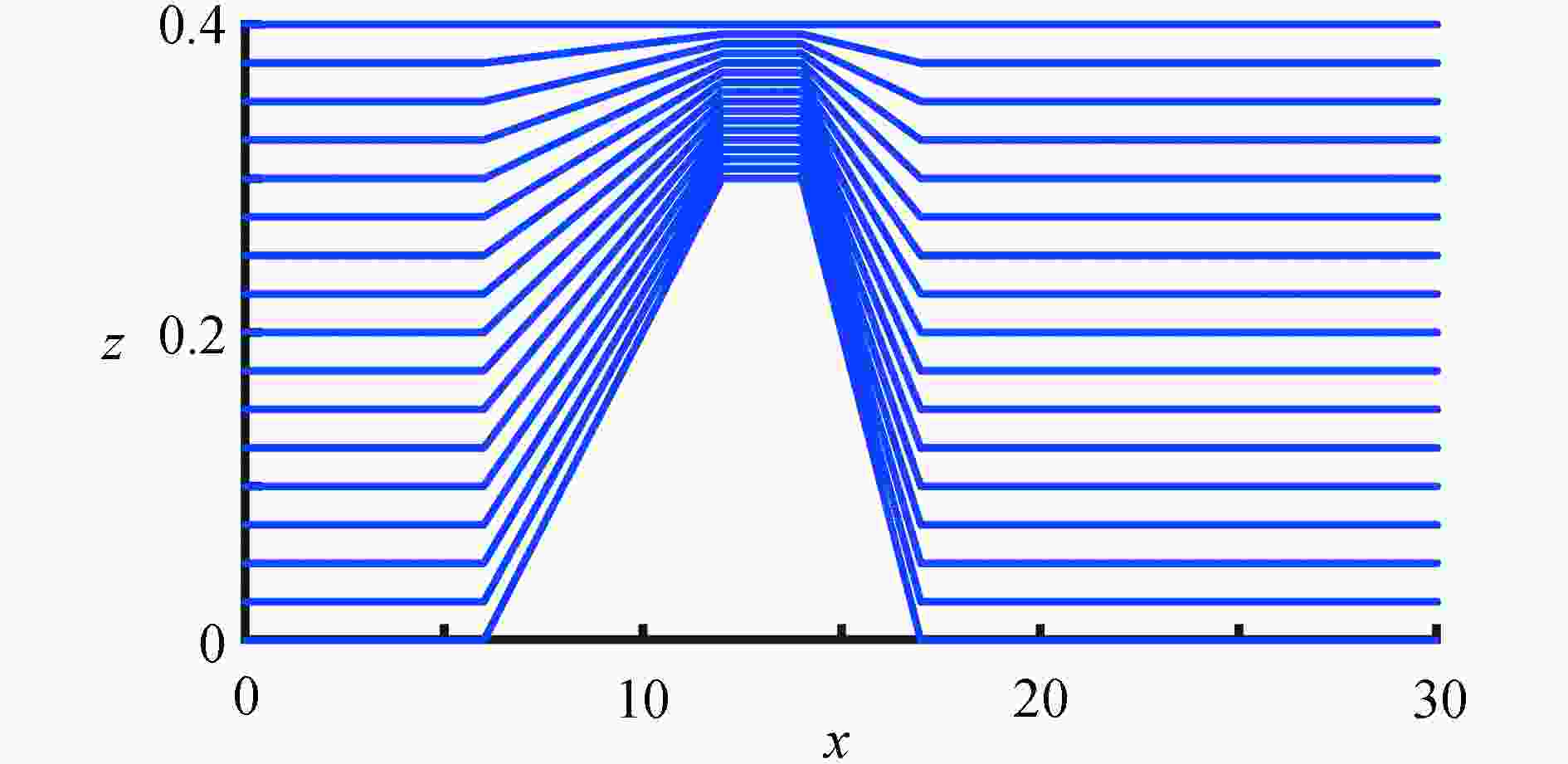
局部边界节点法是一种基于非奇异半解析基函数和移动最小二乘原理的新型无网格配点技术，该方法把每个节点处的未知变量表示为该点对应的局部子域内节点处物理量的线性组合，该文基于局部边界节点法对数值波浪水槽进行了研究。首先，通过基准算例确定了Laplace算子非奇异半解析基函数的合理形状参数值。进一步，基于合理的参数选取，用较少的离散节点即可成功模拟波浪传播行为，将得到的数值结果与其他文献数值结果比较，可以发现局部边界节点法用更少的局部点即可得到较好的数值结果。最后，以保护近海岸建筑物为目标，模拟了水下防波堤对波浪传播的影响。结果表明，当波浪与梯形防波堤发生作用后，波峰变得比较陡峭，而波谷变得相对比较平坦，为近海岸防波堤的相关研究和设计提供了数值参考。
Abstract:The localized boundary knot method (LBKM) is a novel meshless collocation technology based on the non-singular semi-analytical basis functions and the moving least squares theory, and expresses the unknown variable at each knot as a linear combination of physical quantities at nodes inside its corresponding local subdomain. The LBKM was used to study the numerical wave flume. Firstly, the appropriate shape parameters for the non-singular semi-analytical basis functions of the Laplace operator were derived by the benchmark example. Further, the numerical results obtained with fewer nodes and appropriate parameters were in good agreement with the referential results. Finally, the effects of the underwater breakwater on wave propagation were investigated to protect coastal buildings. The results show that, when the wave interacts with the trapezoidal breakwater, the wave crest will become steeper, and the wave trough will become relatively flatter, which provides a numerical reference for the research and design of the coastal breakwater.
-
图 2 在
$x = 4$ 处自由液面高程演化图：(a)不同总节点数；(b)不同时间间隔；(c)不同邻近点数Figure 2. Evolution of free-surface elevation at
$x = 4 :$ (a) different total numbers of nodes; (b) different time increments; (c) different numbers of nearest nodes
图 3 自由表面在4个不同时刻的轮廓
Figure 3. Profiles of free surface along the flume at 4 specific moments
图 4 梯形防波堤数值波浪水槽的示意图
Figure 4. The schematic diagram of the numerical wave flume with a trapezoidal submerged obstacle
图 5 梯形防波堤数值波浪水槽的布点图
Figure 5. Distributions of nodes of the numerical wave flume with a trapezoidal submerged obstacle
图 7 自由液面从
$x = 6$ 到$x = 17$ 在特定时刻的表面轮廓Figure 7. Free surface profiles from
$x = 6$ to$x = 17$ at specific moments表 1 不同形状参数下二阶Stokes波的均方根误差
Table 1. The RMSEs for 2nd-order Stokes waves with different shape parameters
$c$ 0.01 0.1 0.5 1 1.5 2 2.5 3 3.2 εRMSE1 \ $5.23 \times {10^{ - 3}}$ $6.13 \times {10^{ - 4}}$ $5.81 \times {10^{ - 4}}$ $6.16 \times {10^{ - 4}}$ $6.50 \times {10^{ - 4}}$ $6.84 \times {10^{ - 4}}$ $7.19 \times {10^{ - 4}}$ \ 注：“\”表示无法计算，后同。
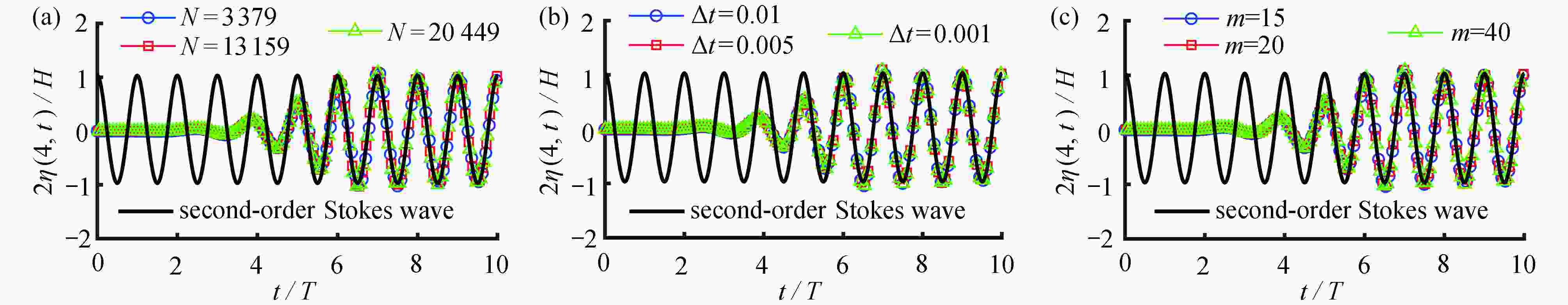
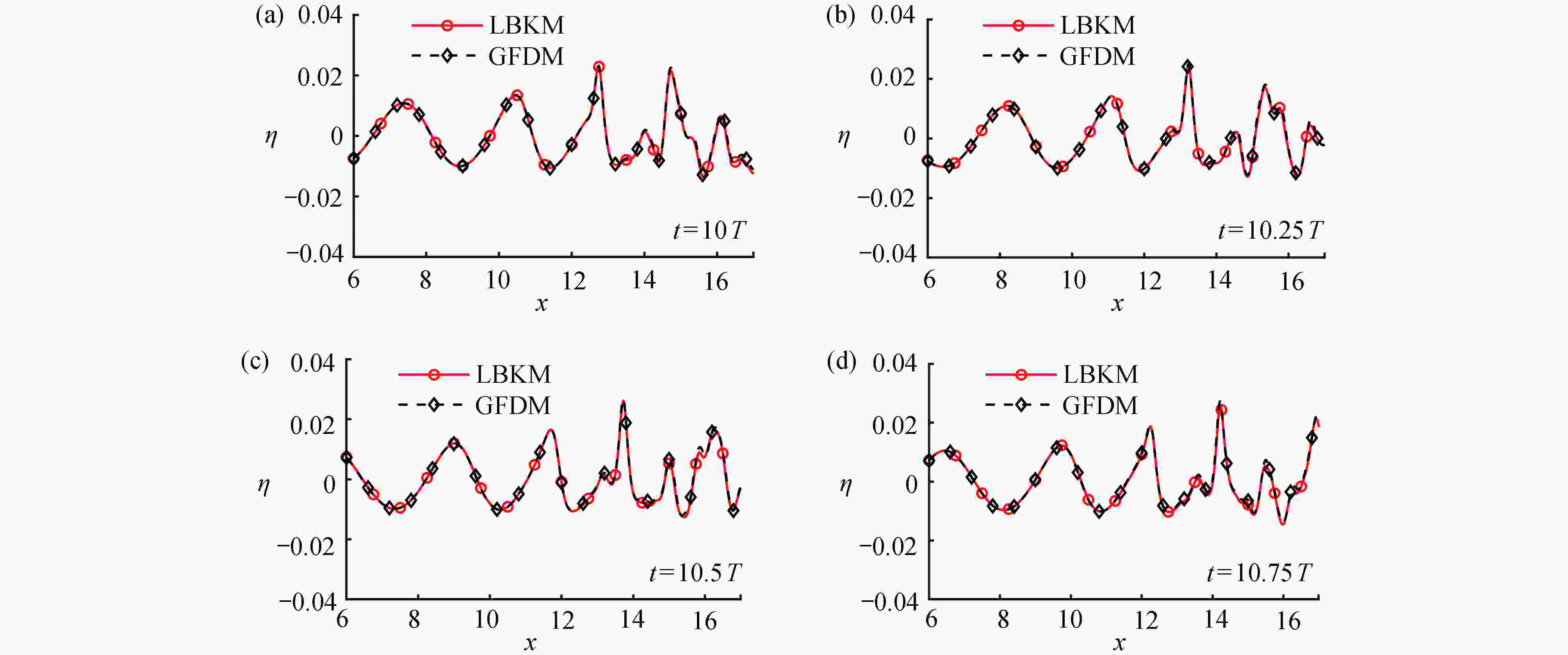
Note: “\” means unable to calculate, the same below.
下载: 导出CSV
表 2 不同总点数下LBKM与GFDM的均方根误差
Table 2. The RMSE1s of LBKM and GFDM under different total numbers of nodes
$N$ 889 3379 13159 20449 LBKM $7.28 \times {10^{ - 4}}$ $6.18 \times {10^{ - 4}}$ $5.81 \times {10^{ - 4}}$ $5.78 \times {10^{ - 4}}$ GFDM $5.98 \times {10^{ - 3}}$ $1.28 \times {10^{ - 3}}$ $4.71 \times {10^{ - 4}}$ $4.63 \times {10^{ - 4}}$
下载: 导出CSV
表 3 不同邻近点数下LBKM与GFDM的均方根误差
Table 3. The RMSE1s of LBKM and GFDM under different numbers of nearest nodes
$m$ 6 10 14 16 18 20 25 30 LBKM $5.04 \times {10^{ - 3}}$ $6.13 \times {10^{ - 4}}$ $5.91 \times {10^{ - 4}}$ $5.85 \times {10^{ - 4}}$ $5.82 \times {10^{ - 4}}$ $5.81 \times {10^{ - 4}}$ $6.53 \times {10^{ - 4}}$ $2.56 \times {10^{ - 3}}$ GFDM \ \ $4.78 \times {10^{ - 4}}$ $4.77 \times {10^{ - 4}}$ $4.75 \times {10^{ - 4}}$ $4.71 \times {10^{ - 4}}$ $2.34 \times {10^{ - 3}}$ $3.58 \times {10^{ - 3}}$
下载: 导出CSV
表 4 不同总点数下
$\phi $ ，${{\partial \phi } / {\partial x}}$ 和${{{\partial ^2}\phi } / {\partial {x^2}}}$ 的均方根误差Table 4. The RMSE2s of
$\phi $ ,${{\partial \phi } / {\partial x}}$ and${{{\partial ^2}\phi } / {\partial {x^2}}}$ under different total numbers of nodes$N$ 889 3379 13159 20449 $\phi $ $4.63 \times {10^{ - 3}}$ $3.85 \times {10^{ - 3}}$ $2.42 \times {10^{ - 3}}$ $2.35 \times {10^{ - 3}}$ ${{\partial \phi }/{\partial x}}$ $1.29 \times {10^{ - 2}}$ $6.65 \times {10^{ - 3}}$ $6.52 \times {10^{ - 3}}$ $5.26 \times {10^{ - 3}}$ ${{{\partial ^2}\phi } / {\partial {x^2}}}$ $3.96 \times {10^{ - 2}}$ $2.86 \times {10^{ - 2}}$ $2.58 \times {10^{ - 2}}$ $1.26 \times {10^{ - 2}}$
下载: 导出CSV
-
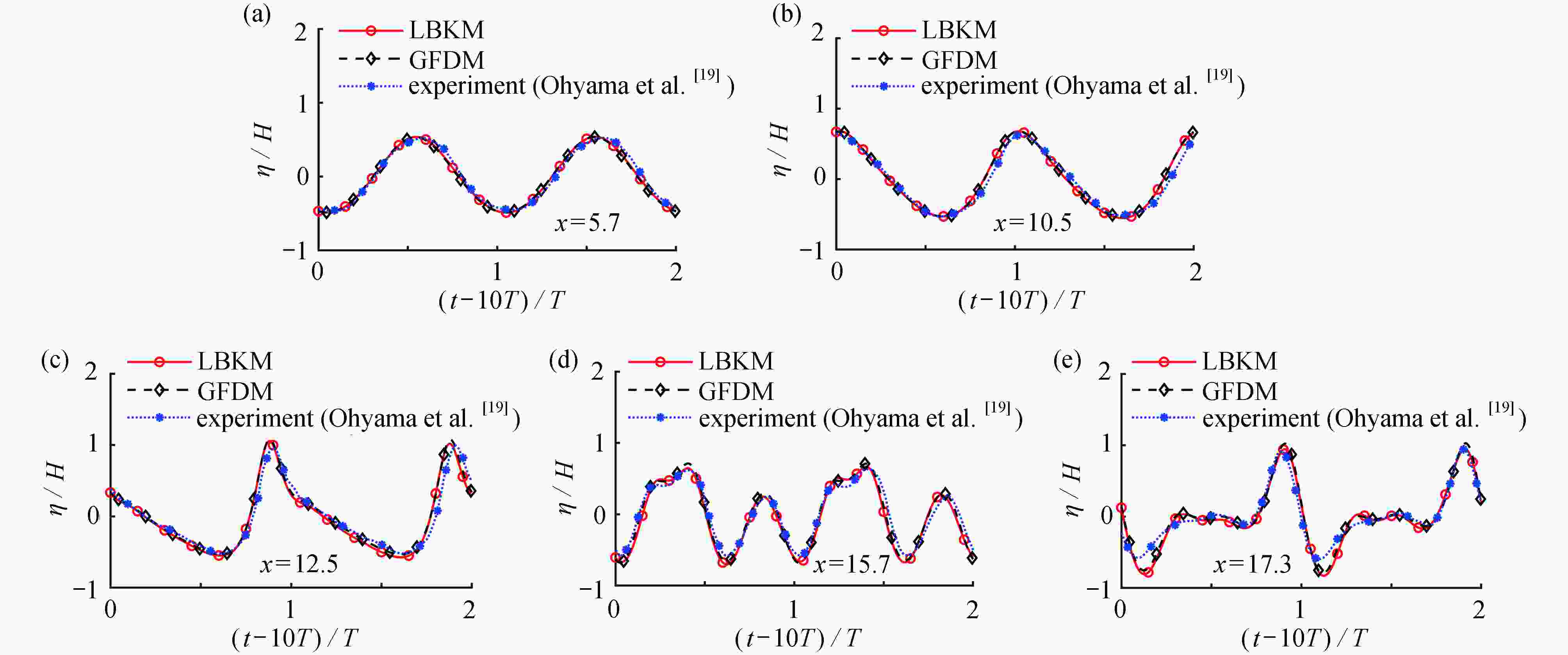
[1] GRILLI S T, SKOURUP J, SVENDSEN I A. An efficient boundary element method for nonlinear water waves[J]. Engineering Analysis With Boundary Elements, 1989, 6(2): 97-107. doi: 10.1016/0955-7997(89)90005-2 [2] 王本龙, 刘桦. 一种适用于非均匀地形的高阶Boussinesq水波模型[J]. 应用数学和力学, 2005, 26(6): 714-722. (WANG Benlong, LIU Hua. Higher order Boussinesq-type equations for water waves on uneven bottom[J]. Applied Mathematics and Mechanics, 2005, 26(6): 714-722.(in Chinese) doi: 10.3321/j.issn:1000-0887.2005.06.013 [3] 王大国, 邹志利, 唐春安. 三维完全非线性波浪水槽的数值模拟[J]. 海洋学报, 2006, 28(4): 138-144. (WANG Daguo, ZOU Zhili, TANG Chunan. Fully nonlinear three-dimensional numerical wave tank simulation[J]. Acta Oceanologica Sinica, 2006, 28(4): 138-144.(in Chinese) [4] 卫志军, 申利敏, 关晖, 等. 拓扑优化技术在抑制流体晃荡中的数值模拟研究[J]. 应用数学和力学, 2021, 42(1): 49-57. (WEI Zhijun, SHEN Limin, GUAN Hui, et al. Numerical simulation of topology optimization technique for tank sloshing suppression[J]. Applied Mathematics and Mechanics, 2021, 42(1): 49-57.(in Chinese) [5] KOO W C, KIM M H. Freely floating-body simulation by a 2D fully nonlinear numerical wave tank[J]. Ocean Engineering, 2004, 31(16): 2011-2046. doi: 10.1016/j.oceaneng.2004.05.003 [6] CHRISTOU M, SWAN C, GUDMESTAD O T. The interaction of surface water waves with submerged breakwaters[J]. Coastal Engineering, 2008, 55(12): 945-958. doi: 10.1016/j.coastaleng.2008.02.014 [7] 黄志涛, 杨瑜, 邵家儒, 等. 罐车防晃结构SPH模拟研究[J]. 应用数学和力学, 2020, 41(7): 760-770. (HUANG Zhitao, YANG Yu, SHAO Jiaru, et al. Numerical simulation of sloshing-mitigating structures in tank trucks with the SPH method[J]. Applied Mathematics and Mechanics, 2020, 41(7): 760-770.(in Chinese) [8] SENTURK U. Modeling nonlinear waves in a numerical wave tank with localized meshless RBF method[J]. Computers and Fluids, 2011, 44(1): 221-228. doi: 10.1016/j.compfluid.2011.01.004 [9] 李珺璞, 傅卓佳, 陈文. 奇异边界法分析含水下障碍物水域中的水波传播问题[J]. 应用数学和力学, 2015, 36(10): 1035-1044. (LI Junpu, FU Zhuojia, CHEN Wen. The singular boundary method for obliquely incident water wave passing a submerged breakwater[J]. Applied Mathematics and Mechanics, 2015, 36(10): 1035-1044.(in Chinese) doi: 10.3879/j.issn.1000-0887.2015.10.003 [10] ZHANG T, REN Y F, FAN C M, et al. Simulation of two-dimensional sloshing phenomenon by generalized finite difference method[J]. Engineering Analysis With Boundary Elements, 2016, 63: 82-91. doi: 10.1016/j.enganabound.2015.11.008 [11] ZHANG T, REN Y F, YANG Z Q, et al. Application of generalized finite difference method to propagation of nonlinear water waves in numerical wave flume[J]. Ocean Engineering, 2016, 123: 278-290. doi: 10.1016/j.oceaneng.2016.07.038 [12] HUANG J, CHU C N, FAN C M, et al. On the propagation of nonlinear water waves in a three-dimensional numerical wave flume using the generalized finite difference method[J]. Engineering Analysis With Boundary Elements, 2020, 119: 225-234. doi: 10.1016/j.enganabound.2020.07.020 [13] ZHANG Y O, XIONG A K. A particle method based on a generalized finite difference scheme to solve weakly compressible viscous flow problems[J]. Symmetry, 2019, 11(9): 1086. doi: 10.3390/sym11091086 [14] CHEN W, TANAKA M. A meshless, integration-free, and boundary-only RBF technique[J]. Computers & Mathematics With Applications, 2002, 43(3/5): 379-391. [15] FU Z J, XI Q, CHEN W, et al. A boundary-type meshless solver for transient heat conduction analysis of slender functionally graded materials with exponential variations[J]. Computers & Mathematics With Applications, 2018, 76(4): 760-773. [16] WANG F J, GU Y, QU W Z, et al. Localized boundary knot method and its application to large-scale acoustic problems[J]. Computer Methods in Applied Mechanics and Engineering, 2020, 361: 112729. doi: 10.1016/j.cma.2019.112729 [17] XIONG J G, WEN J C, LIU Y C. Localized boundary knot method for solving two-dimensional Laplace and bi-harmonic equations[J]. Mathematics, 2020, 8(8): 1218. doi: 10.3390/math8081218 [18] RICCHIUTO M, ABGRALL R. Explicit Runge-Kutta residual distribution schemes for time dependent problems: second order case[J]. Journal of Computational Physics, 2010, 229(16): 5653-5691. doi: 10.1016/j.jcp.2010.04.002 [19] OHYAMA T, BEJI S, NADAOKA K, et al. Experimental verification of numerical model for nonlinear wave evolutions[J]. Journal of Waterway, Port, Coastal and Ocean Engineering, 1994, 120(6): 637-644. doi: 10.1061/(ASCE)0733-950X(1994)120:6(637) -
计量
- 文章访问数: 691
- HTML全文浏览量: 307
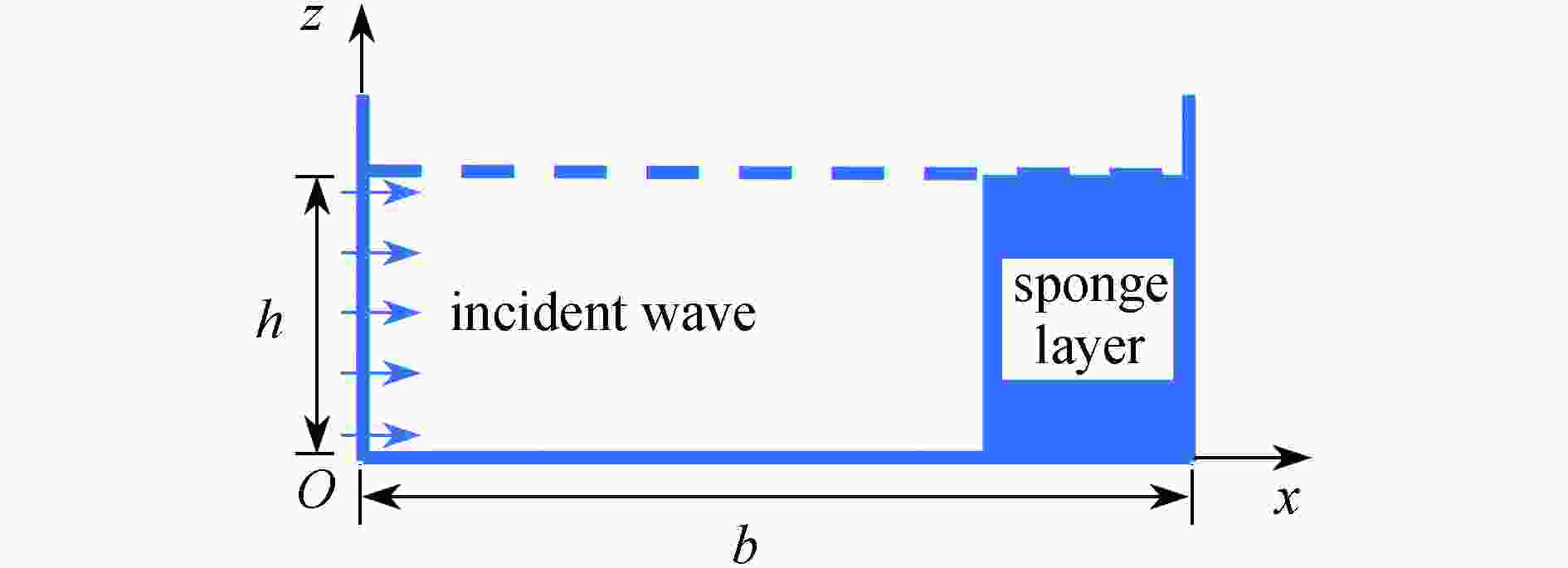
- PDF下载量: 71
- 被引次数: 0
渝公网安备50010802005915号