Dynamics Analysis of Cannibalistic Model With Density Dependence in Mature Stage
-
摘要:
在考虑成熟阶段具有密度制约的基础上，建立了一类具有卵-成熟阶段的同类相食模型。该文从两个方面讨论了模型的动力学性态：当种群不存在同类相食时，构造Lyapunov函数证明平衡点的全局渐近稳定性；当种群存在同类相食时，利用中心流形定理证明同类相食使模型产生鞍结点分支，通过构造Dulac函数说明在二维自治系统中不存在极限环，得到了平衡点的全局稳定性。最后，利用数值模拟验证了所得相应结果的正确性。
Abstract:In view of the density dependence of mature individuals, a two-stage cannibalistic model with the egg-to-maturity stage was established. The dynamic behaviors of the model were discussed from two aspects. In the case without cannibalism, the global asymptotic stability of the equilibrium points was proved through construction of the Lyapunov function, while in the case with cannibalism, the existence of saddle-node bifurcation due to cannibalism was proved with the center manifold theorem. Through construction of the Dulac function, nonexistence of the limit cycle in the two-dimensional autonomous system was elucidated, and therefore, the global stability of the equilibrium points was obtained. Finally, the theoretical results were verified through numerical simulation.
-
Key words:
- cannibalism /
- saddle-node bifurcation /
- density dependence /
- Dulac function
-
图 1 模型(1)存在种群灭绝平衡点时的动力学性态
Figure 1. The model (1) dynamics with a population extinction equilibrium point
图 2 模型(1)在
$ d \lt r $ 时存在唯一种群存活平衡点的动力学性态Figure 2. The model (1) dynamics with a unique population survival equilibrium point for
$ d \lt r $ 
图 3 模型(1)存在两个种群存活平衡点的动力学性态
Figure 3. The model (1) dynamics with 2 population survival equilibrium points
-
[1] 祖力, 黄冬冬, 柳扬. 捕食者和食饵均带有扩散的随机捕食-食饵模型动力学分析[J]. 应用数学和力学, 2017, 38(3): 355-368ZU Li, HUANG Dongdong, LIU Yang. Dynamics of dual-dispersal predator-prey systems under stochastic perturbations[J]. Applied Mathematics and Mechanics, 2017, 38(3): 355-368.(in Chinese) [2] 王小娥, 蔺小林, 李建全. 具有Holling IV型功能反应捕食系统的状态反馈控制[J]. 应用数学和力学, 2020, 41(12): 1369-1380WANG Xiaoe, LIN Xiaolin, LI Jianquan. State feedback control of predator-prey systems with Holling IV functional responses[J]. Applied Mathematics and Mechanics, 2020, 41(12): 1369-1380.(in Chinese) [3] 陈乾君, 蒋媛, 刘子建, 等. 具有Gilpin-Ayala增长的随机捕食-食饵模型的动力学行为[J]. 应用数学和力学, 2022, 43(4): 453-468CHEN Qianjun, JIANG Yuan, LIU Zijian, et al. Dynamic behavior of a stochastic predator prey model with the Gilpin-Ayala growth[J]. Applied Mathematics and Mechanics, 2022, 43(4): 453-468.(in Chinese) [4] 黄大明, 张天曦, 李淑芬, 等. 自然种群中的同类相食[J]. 中国科技纵横, 2019(7): 252-256 doi: 10.3969/j.issn.1671-2064.2019.07.116HUANG Daming, ZHANG Tianxi, LI Shufen, et al. Cannibalism in natural populations[J]. China Science & Technology Overview, 2019(7): 252-256.(in Chinese) doi: 10.3969/j.issn.1671-2064.2019.07.116 [5] CUSHING J M, HENSON S M, HAYWARD J L. An evolutionary game-theoretic model of cannibalism[J]. Natural Resource Modeling, 2015, 28(4): 497-521. doi: 10.1111/nrm.12079 [6] CUSHING J M. A simple model of cannibalism[J]. Mathematical Biosciences, 1991, 107(1): 47-71. doi: 10.1016/0025-5564(91)90071-P [7] RICHARDSON M L, MITCHELL R F, REAGEL P F, et al. Causes and consequences of cannibalism in noncarnivorous insects[J]. Annual Review of Entomology, 2015, 55(1): 39-53. [8] GABRIEL W. Overcoming food limitation by cannibalism: a model study on cyclopoids[C]//Ergebnisse der Limnologie Advances in Iimnology. Stuttgart: E. Schweizerbart’sche Verlagsbuchhandlung (Nägele u. Obermiller), 1985: 373-381. [9] VAN DEN BOSCH F, DE ROOS A M, GABRIEL W. Cannibalism as a life boat mechanism[J]. Journal of Mathematical Biology, 1988, 26(6): 619-633. doi: 10.1007/BF00276144 [10] VAN DEN BOSCH F, GABRIEL W. Cannibalism in an age-structured predator-prey system[J]. Bulletin of Mathematical Biology, 1997, 59(3): 551-567. doi: 10.1007/BF02459465 [11] WIKAN A, EIDE A. An analysis of a nonlinear stage-structured cannibalism model with application to the northeast arctic cod stock[J]. Bulletin of Mathematical Biology, 2004, 66(6): 1685-1704. doi: 10.1016/j.bulm.2004.03.005 [12] CHAKRABORTY K, DAS K, KAR T K. Combined harvesting of a stage structured prey-predator model incorporating cannibalism in competitive environment[J]. Comptes Rendus Biologies, 2013, 336(1): 34-45. doi: 10.1016/j.crvi.2013.01.002 [13] BISWAS S, CHATTERJEE S, CHDATTOPADHYAY J. Cannibalism may control disease in predator population: result drawn from a model based study[J]. Mathematical Methods in the Applied Sciences, 2015, 38(11): 2272-2290. doi: 10.1002/mma.3220 [14] ZHANG F Q, CHEN Y M, LI J Q. Dynamical analysis of a stage-structured predator-prey model with cannibalism[J]. Mathematical Biosciences, 2019, 307: 33-41. doi: 10.1016/j.mbs.2018.11.004 [15] CHEN M J, FU S M, YANG X L. Global behavior of solutions in a predator-prey cross-diffusion model with cannibalism[J]. Complexity, 2020, 2020: 1265798. [16] 朱雪, 蔺小林, 李建全. 一类具有两阶段结构同类相食模型的动力学分析[J]. 工程数学学报, 2021, 38(2): 214-228 doi: 10.3969/j.issn.1005-3085.2021.02.006ZHU Xue, LIN Xiaolin, LI Jianquan. A dynamics analysis of cannibalism model with two-stage structure[J]. Chinese Journal of Engineering Mathematics, 2021, 38(2): 214-228.(in Chinese) doi: 10.3969/j.issn.1005-3085.2021.02.006 [17] 马杏园, 邱志鹏. 一类具有同类相食的昆虫传染病模型分析[J]. 山西大学学报(自然科学版), 2022, 45(2): 348-355MA Xingyuan, QIU Zhipeng. Analysis of an insect epidemic model with cannibalism[J]. Journal of Shanxi University (Natural Science Edition) , 2022, 45(2): 348-355.(in Chinese) [18] KANG Y, RODRIGUEZ-RODRIGUEZ M, EVILSIZOR S. Ecological and evolutionary dynamics of two-stage models of social insects with egg cannibalism[J]. Journal of Mathematical Analysis and Applications, 2015, 430(1): 324-353. doi: 10.1016/j.jmaa.2015.04.079 [19] NAKAMURA K, HASAN N, ABBAS I, et al. Generation cycles in Indonesian lady beetle populations may occur as a result of cannibalism[J]. Proceedings of the Royal Society B: Biological Sciences, 2004, 271: S501-S504. doi: 10.1098/rspb.2003.2608 [20] 陈兰荪, 王东达, 杨启昌. 阶段结构种群动力学模型[J]. 北华大学学报(自然科学版), 2000, 1(3): 185-191CHEN Lansun, WANG Dongda, YANG Qichang. The models of stage-structured population dynamics[J]. Journal of Beihua University (Natural Science) , 2000, 1(3): 185-191.(in Chinese) [21] 赵甜, 张凤琴, 李建全. 同类相食对两阶段结构种群模型的动力学影响[J]. 数学的实践与认识, 2017, 47(20): 147-154ZHAO Tian, ZHANG Fengqin, LI Jianquan. Effect of cannibalism on dynamics of a population model with two-stage structure[J]. Mathematics in Practice and Theory, 2017, 47(20): 147-154.(in Chinese) [22] 李静, 孙桂全, 靳祯. 种内竞争时滞对植被周期振荡模式的影响研究[J]. 应用数学和力学, 2022, 43(6): 669-681LI Jing, SUN Guiquan, JIN Zhen. Effect of intraspecific competition delay on vegetation periodic oscillation pattern[J]. Applied Mathematics and Mechanics, 2022, 43(6): 669-681.(in Chinese) -
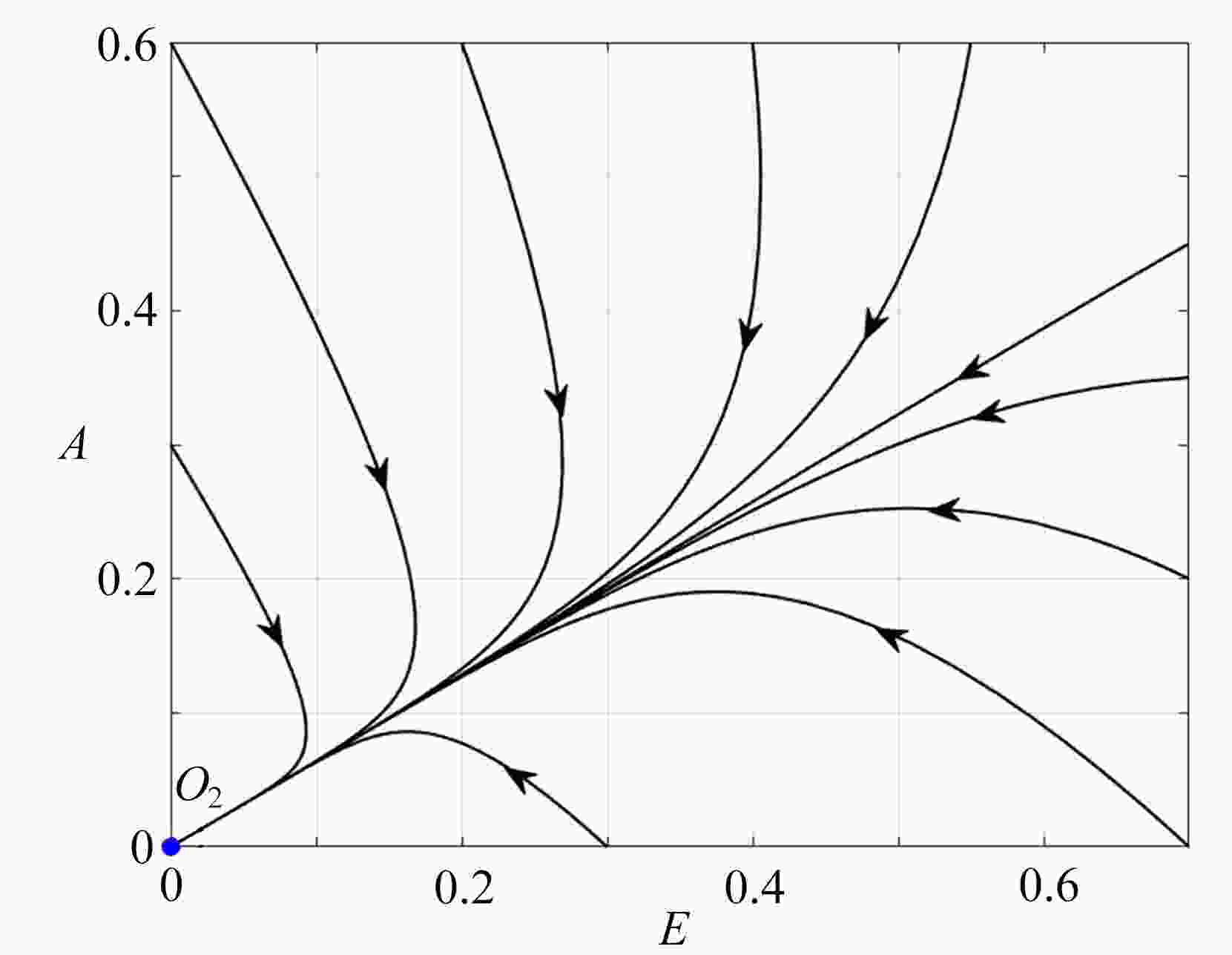
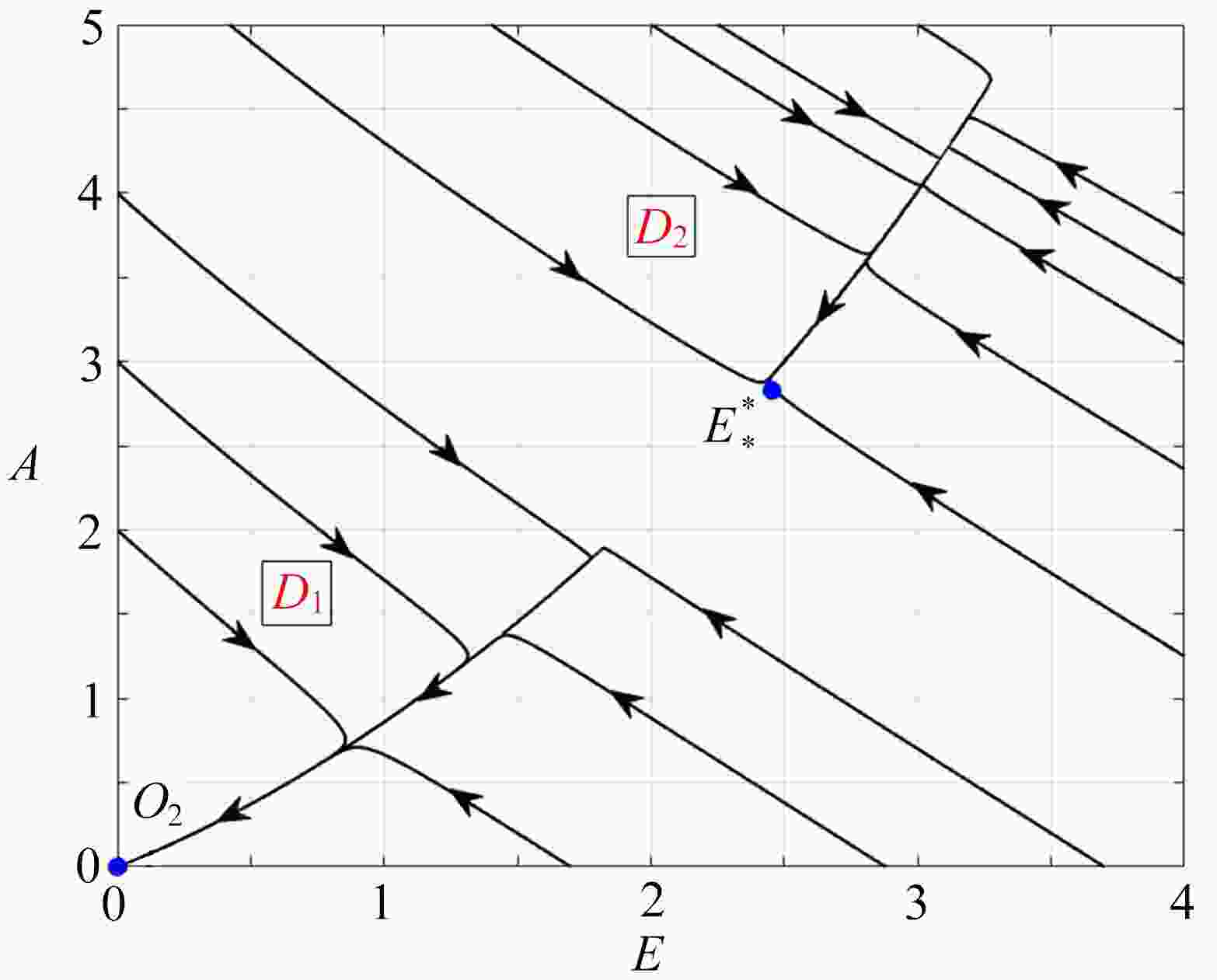
下载:
计量
- 文章访问数: 488
- HTML全文浏览量: 192
- PDF下载量: 63
- 被引次数: 0
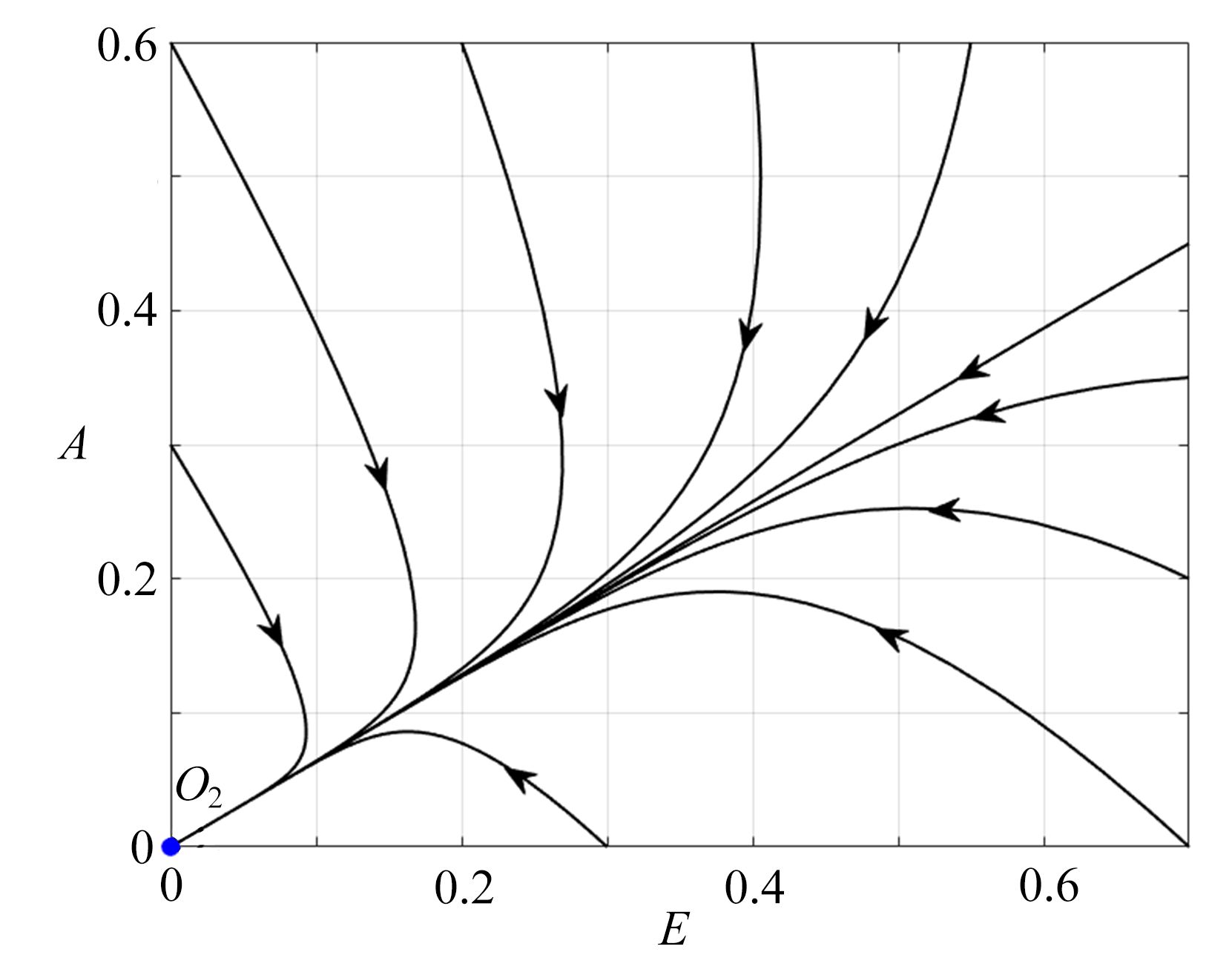
渝公网安备50010802005915号