弹着点对钢筋混凝土侵彻深度的影响

黄成龙; 陈叶青; 李述涛; 张生; 王振清

doi:10.21656/1000-0887.440016

弹着点对钢筋混凝土侵彻深度的影响

doi: 10.21656/1000-0887.440016

黄成龙^{1, 2,},
陈叶青^{1, 2},
李述涛²,
张生^{1, 2},
王振清^1, ,

1.
哈尔滨工程大学航天与建筑工程学院，哈尔滨 150001
2.
军事科学院国防工程研究院，北京 100036

(我刊编委王振清来稿)

基金项目:

国家自然科学基金项目 11532013

国家自然科学基金项目 11872157

详细信息

作者简介:
黄成龙(1997—)，男，博士生(E-mail: huangchenglong@hrbeu.edu.cn)

通讯作者:
王振清(1962—)，男，教授，博士，博士生导师(通讯作者. E-mail: wangzhenqing@hrbeu.edu.cn)

中图分类号: O385
计量
- 文章访问数: 500
- HTML全文浏览量: 120
- PDF下载量: 51
- 被引次数: 0
出版历程
- 收稿日期: 2023-01-18
- 修回日期: 2023-07-05
- 刊出日期: 2023-09-01

Influences of Impact Points on the Penetration Depth of Reinforced Concrete

HUANG Chenglong^{1, 2
,},
CHEN Yeqing^{1, 2},
LI Shutao²,
ZHANG Sheng^{1, 2},
WANG Zhenqing^{1
, ,}

1.
College of Aerospace and Civil Engineering, Harbin Engineering University, Harbin 150001, P.R.China
2.
Institute of Defense Engineering, Academy of Military Sciences, Beijing 100036, P.R.China

(Contributed by WANG Zhenqing, M. AMM Editorial Board)

摘要

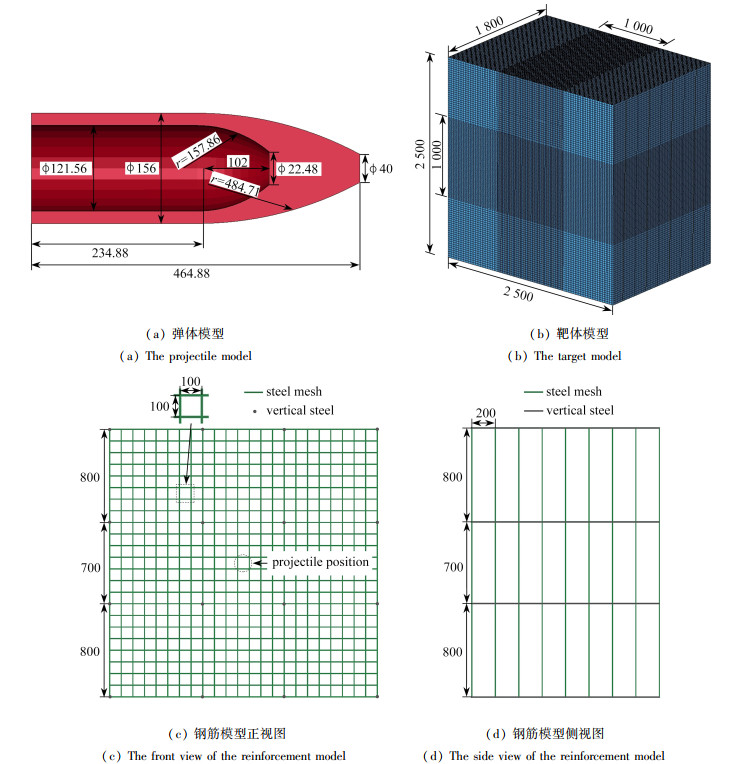
摘要: 在弹体侵彻钢筋混凝土研究领域，侵彻深度的离散性普遍存在于试验和经验公式中，弹着点位置的不同是造成此离散性的主要原因之一. 为探究由弹着点位置造成的侵彻深度离散性并揭示其机理，参照公开发表的侵彻试验，建立了三种典型弹着点位置的有限元模型，对比分析出了三种典型弹着点位置侵彻过程差异的主要原因，依据数值计算结果归纳了表征侵彻深度离散性的表达式，提出了弹体侵彻钢筋混凝土侵彻深度是一个范围值的基本思想，并对表达式进行了初步验证. 结果表明，造成侵彻深度离散性的主要因素是弹体撞击钢筋的数目和弹体接触钢筋的持续时间，此离散性随着弹径与钢筋网眼尺寸比值的增大而减小.
- 弹着点 /
- 弹体侵彻 /
- 钢筋混凝土 /
- 侵彻深度
Abstract: In the research field of projectile penetration into reinforced concrete, the penetration depth discreteness generally exists in experiments and empirical formulas, and the difference of impact positions is one of the main reasons for this discreteness. To explore the penetration depth discreteness caused by the impact position difference and reveal its mechanism, the finite element models of 3 typical impact positions were established with reference to a published penetration test. The main reasons for the differences in the penetration processes of 3 typical impact positions were compared and analyzed. Based on the numerical calculation results, the expression characterizing the discreteness of penetration depth was summarized. The results show that, the penetration depth of the projectile impacting reinforced concrete is a range value. The expression was preliminarily verified. The main factors causing the penetration depth discreteness are the number of rebars hit by the projectile and the duration of contact with steel bars. This discreteness decreases with the ratio of the projectile diameter to the mesh size of rebars.
- impact point /
- projectile penetration /
- reinforced concrete /
- penetration depth
edited-by

edited-by
注释:

1) (我刊编委王振清来稿)

HTML全文

图 1 全尺寸模型(单位：mm)

注为了解释图中的颜色，读者可以参考本文的电子网页版本，后同.

Figure 1. The full-size model(unit: mm)

下载: 全尺寸图片幻灯片

图 2 弹塑性硬化模型应力-应变图

Figure 2. The stress-strain relationship of the elastoplastic hardening model

下载: 全尺寸图片幻灯片

图 3 靶体损伤情况对比

Figure 3. Comparison of target damages

下载: 全尺寸图片幻灯片

图 4 数值模拟侵彻深度时程图

Figure 4. The penetration depth time history of the numerical simulation

下载: 全尺寸图片幻灯片

图 5 全区域精细化网格靶体(单位: mm)

Figure 5. The target model with global fine grids(unit: mm)

下载: 全尺寸图片幻灯片

图 6 三种典型弹着点位置示意图

Figure 6. Schematic diagram of 3 typical impact point positions

下载: 全尺寸图片幻灯片

图 7 三种典型弹着点位置时程图对比

Figure 7. Time history comparison of 3 typical impact point positions

下载: 全尺寸图片幻灯片

图 8 3.1 ms前弹体加速度时程图

Figure 8. Projectile acceleration time histories before 3.1 ms

下载: 全尺寸图片幻灯片

图 9 钢筋交叉点情况下侵彻过程模拟图

Figure 9. Simulation diagrams of the penetration process at the rebar crossing point

下载: 全尺寸图片幻灯片

图 10 钢筋网眼中点情况下侵彻过程模拟图

Figure 10. Simulation diagrams of the penetration process at the rebar grid midpoint

下载: 全尺寸图片幻灯片

图 11 钢筋侧边中点情况下侵彻过程模拟图

Figure 11. Simulation diagrams of the penetration process at the rebar side midpoint

下载: 全尺寸图片幻灯片

图 12 侵彻深度与d/D的关系

Figure 12. Relationships between the penetration depth and d/D

下载: 全尺寸图片幻灯片

图 13 无量纲表达式拟合曲线

Figure 13. The fitting curve of the dimensionless expression

下载: 全尺寸图片幻灯片

图 14 归一化侵彻深度与d/D的关系

Figure 14. Relationships between the normalized

下载: 全尺寸图片幻灯片

图 15 归一化侵彻深度关于d/D的拟合曲线

Figure 15. Fitting curves of the normalized penetration penetration depth and d/D depth with respect to d/D

下载: 全尺寸图片幻灯片

表 1 弹体及钢筋材料模型参数

Table 1. Material model parameters of the projectile and the reinforcement

material name	ρ/(kg·m^-3)	E/Pa	μ	σ₀/Pa	E_t/Pa	β	C/ s^-1	P	failure strain ε_F
projectile	7.91×10³	2.1×10¹¹	0.30	-	-	-	-	-	-
reinforcement	7.80×10³	2.0×10¹¹	0.29	3.45×10⁸	2×10⁹	0			0.8

下载: 导出CSV

表 2 混凝土材料模型参数

Table 2. Material model parameters of concrete

material name	ρ/(kg·m^-3)	μ	F_t/Pa	A₀/Pa	α	β
concrete	2.44×10³	0.2	4×10⁶	-4.8×10⁷	39.37	1.45×10^-4

下载: 导出CSV

表 3 侵彻深度计算结果

Table 3. Calculation results of the penetration depth

impact point position	rebar grid midpoint	rebar crossing point	rebar side midpoint
h/mm	1 113	1 149	1 117

下载: 导出CSV

表 4 各工况数值计算结果

Table 4. Numerical calculation results of each working condition

d/mm	D/mm	d/D	μ/%	h/mm
d/mm	D/mm	d/D	μ/%	rebar grid midpoint	rebar crossing point	average value	difference value
156	200	0.78	0.36	1 490	1 230	1 360	260
156	150	1.04	0.48	1 250	1 160	1 205	90
156	100	1.56	0.71	1 113	1 149	1 131	-36
156	80	1.95	0.83	978	1 030	1 004	-52
156	70	2.23	0.94	963	952	957.5	11
156	60	2.60	1.12	934	907	920.5	27
156	50	3.12	1.35	869	874	871.5	-5
156	40	3.90	1.69	843	863	853	-20
156	34	4.59	1.98	815	799	807	16
156	30	5.20	2.21	763	749	756	14

下载: 导出CSV

表 5 数值模拟和表达式的计算结果

Table 5. Calculation results of the numerical simulation and the expression

D/mm	d/D	h_M/mm		error δ_M/%	h_C/mm		error δ_C/%
D/mm	d/D	simulation	expression	error δ_M/%	simulation	expression	error δ_C/%
172	0.91	1 291	1 363	5.58	1 199	1 201	0.17
90	1.73	1 018	1 031	1.28	1 081	1 084	0.28
44	3.55	865	847	2.08	866	859	0.81

下载: 导出CSV

参考文献(27)

[1]	YOUNG C W. Penetration equations: SAND97-2426[R]. Albuquerque, NM, USA, 1997.
[2]	BERNARD R S, CREIGHTON D C. Projectile penetration in soil and rock: analysis for non-normal impact[R]. Vicksburg, USA, 1979.
[3]	BERNARD R S. Depth and motion prediction for earth penetrators[R]. Vicksburg, USA, 1978.
[4]	BERNARD R S. Empirical analysis of projectile penetration in rock[R]. Vicksburg, USA, 1977.
[5]	FORRESTAL M J, ALTMAN B S, CARGILE J D, et al. An empirical equation for penetration depth of ogive-nose projectiles into concrete targets[J]. International Journal of Impact Engineering, 1994, 15(4): 395-405. doi: 10.1016/0734-743X(94)80024-4
[6]	National Defence Research Committee. Effects of impact and explosion[R]. Washington DC, USA, 1946.
[7]	Department of the Army. Fundamentals of protective design for conventional weapons: TM 5-855-1[R]. Washington DC, USA, 1986.
[8]	王安宝, 邓国强, 杨秀敏, 等. 一个新的通用型侵彻深度计算公式[J]. 土木工程学报, 2021, 54(10): 36-46. https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC202110005.htm WANG Anbao, DENG Guoqiang, YANG Xiumin, et al. A new general formula for calculating penetration depth[J]. China Civil Engineering Journal, 2021, 54(10): 36-46. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC202110005.htm
[9]	任辉启, 穆朝民, 刘瑞朝, 等. 精确制导武器侵彻效应与工程防护[M]. 北京: 科学出版社, 2016. REN Huiqi, MU Chaomin, LIU Ruichao, et al. Penetration Effect and Engineering Protection of Precision Guided Weapons[M]. Beijing: Science Press, 2016. (in Chinese)
[10]	刘云飞, 王天运, 蒋沧如. 弹体侵彻混凝土深度计算公式分析[J]. 武汉理工大学学报, 2004, 26(1): 49-52. https://www.cnki.com.cn/Article/CJFDTOTAL-WHGY200401014.htm LIU Yunfei, WANG Tianyun, JIANG Cangru. Analysis on depth calculation of projectiles penetration into concrete[J]. Journal of Wuhan University of Technology, 2004, 26(1): 49-52. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-WHGY200401014.htm
[11]	周宁, 任辉启, 沈兆武, 等. 卵形头部弹丸侵彻钢筋混凝土的工程解析模型[J]. 振动与冲击, 2007, 26(4): 73-76. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ200704016.htm ZHOU Ning, REN Huiqi, SHEN Zhaowu, et al. Engineering analytical model for ogive-nose projectiles to penetrate into semi-infinite reinforced concrete targets[J]. Journal of Vibration and Shock, 2007, 26(4): 73-76. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ200704016.htm
[12]	欧阳春, 赵国志, 杜中华, 等. 弹丸垂直侵彻钢筋混凝土介质的工程解析模型[J]. 爆炸与冲击, 2004, 24(3): 273-277. https://www.cnki.com.cn/Article/CJFDTOTAL-BZCJ200403013.htm OUYANG Chun, ZHAO Guozhi, DU Zhonghua, et al. An engineering analytical model for projectiles to penetrate normally into semi-infinite reinforced concrete targets[J]. Explosion and Shock Waves, 2004, 24(3): 273-277. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-BZCJ200403013.htm
[13]	穆朝民, 任辉启. 弹丸对钢筋混凝土中钢筋交汇处侵彻效应研究[J]. 高压物理学报, 2010, 24(5): 351-358. https://www.cnki.com.cn/Article/CJFDTOTAL-GYWL201005006.htm MU Chaoming, REN Huiqi. Research on the effect of the projectile penetrating into the reinforced concrete targets at the intersection of the steel bar[J]. Chinese Journal of High Pressure Physics, 2010, 24(5): 351-358. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GYWL201005006.htm
[14]	楼建锋, 王政, 朱建士, 等. 含筋率和弹着点对钢筋混凝土抗侵彻性能的影响[J]. 爆炸与冲击, 2010, 30(2): 178-182. https://www.cnki.com.cn/Article/CJFDTOTAL-BZCJ201002013.htm LOU Jianfeng, WANG Zheng, ZHU Jianshi, et al. Effects of reinforcement ratio and impact position on anti-penetration properties of reinforced concrete[J]. Explosion and Shock Waves, 2010, 30(2): 178-182. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-BZCJ201002013.htm
[15]	孙其然, 李芮宇, 赵亚运, 等. HJC模型模拟钢筋混凝土侵彻实验的参数研究[J]. 工程力学, 2016, 33(8): 248-256. https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201608032.htm SUN Qiran, LI Ruiyu, ZHAO Yayun, et al. Investigation on parameters of HJC model applied to simulate perforation experiments of reinforced concrete[J]. Engineering Mechanics, 2016, 33(8): 248-256. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201608032.htm
[16]	程毅, 刘军, 刘晓峰, 等. 垂直侵彻下钢筋混凝土靶抗侵彻性能的理论与数值分析[J]. 科学技术与工程, 2019, 19(1): 97-103. https://www.cnki.com.cn/Article/CJFDTOTAL-KXJS201901015.htm CHENG Yi, LIU Jun, LIU Xiaofeng, et al. Theoretical and numerical analysis on anti-penetration property of reinforced concrete target under normal penetration[J]. Science Technology and Engineering, 2019, 19(1): 97-103. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-KXJS201901015.htm
[17]	武海军, 张爽, 黄风雷. 钢筋混凝土靶的侵彻与贯穿研究进展[J]. 兵工学报, 2018, 39(1): 182-208. https://www.cnki.com.cn/Article/CJFDTOTAL-BIGO201801020.htm WU Haijun, ZHANG Shuang, HUANG Fenglei. Research progress in penetration/perforation into reinforced concrete targets[J]. Acta Armamentarii, 2018, 39(1): 182-208. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-BIGO201801020.htm
[18]	邓勇军, 陈小伟, 钟卫洲, 等. 弹体正侵彻钢筋混凝土靶的试验及数值模拟研究[J]. 爆炸与冲击, 2020, 40(2): 26-36. https://www.cnki.com.cn/Article/CJFDTOTAL-BZCJ202002003.htm DENG Yongjun, CHEN Xiaowei, ZHONG Weizhou, et al. Experimental and numerical study on normal penetration of a projectile into a reinforced concrete target[J]. Explosion and Shock Waves, 2020, 40(2): 26-36. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-BZCJ202002003.htm
[19]	CHEN X W, LI Q M. Transition from nondeformable projectile penetration to semihydrodynamic penetration[J]. Journal of Engineering Mechanics, 2004, 130(1): 123-127.
[20]	张涛, 方秦, 吴昊, 等. 飞机撞击核安全壳不同位置破坏效应的数值模拟[J]. 应用数学和力学, 2015, 36(S1): 107-116. https://www.cnki.com.cn/Article/CJFDTOTAL-YYSX2015S1015.htm ZHANG Tao, FANG Qin, WU Hao, et al. Numerical simulation on the response and damage of nuclear containment under the different aircraft impact positons[J]. Applied Mathematics and Mechanics, 2020, 36(S1): 107-116. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YYSX2015S1015.htm
[21]	MALVAR L J, CRAWFORD J E, WESEVICH J W, et al. A plasticity concrete material model for DYNA3D[J]. International Journal of Impact Engineering, 1997, 19(9/10): 847-873.
[22]	门建兵, 隋树元, 蒋建伟, 等. 网格对混凝土侵彻数值模拟的影响[J]. 北京理工大学学报, 2005, 25(8): 659-662. https://www.cnki.com.cn/Article/CJFDTOTAL-BJLG200508000.htm MEN Jianbing, SUI Shuyuan, JIANG Jianwei, et al. Mesh dependency for numerical simulation of concrete penetration[J]. Journal of Beijing Institute of Technology, 2005, 25(8): 659-662. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-BJLG200508000.htm
[23]	辛春亮, 薛再清, 涂建, 等. 有限元分析常用材料参数手册[M]. 北京: 机械工业出版社, 2019. XIN Chunliang, XUE Zaiqing, TU Jian, et al. Handbook of Material Parameters Commonly Used in Finite Element Analysis[M]. Beijing: China Machine Press, 2019. (in Chinese)
[24]	赵均海, 孙珊珊, 党会学, 等. 钢管混凝土柱抗爆性能数值模拟与实验验证[J]. 应用数学和力学, 2020, 41(9): 943-955. doi: 10.21656/1000-0887.400207 ZHAO Junhai, SUN Shanshan, DANG Huixue, et al. Numerical simulation and test validation for concreted filled steel tube columns under blast loading[J]. Applied Mathematics and Mechanics, 2020, 41(9): 943-955. (in Chinese) doi: 10.21656/1000-0887.400207
[25]	MALVAR L J, SIMONS D. Concrete material modeling in explicit computations[C]//Workshop on Recent Advances in Computational Structural Dynamics and High Performance Computing. Vicksburg, MS: USAE Waterways Experiment Station, 1996.
[26]	熊益波. LS-DYNA中简单输入混凝土模型适用性分析[C]//第十一届全国冲击动力学学术会议论文集. 西安: 西北核技术研究所, 2013. XIONG Yibo. Applicability analysis of simple input concrete models in LS-DYNA[C]//Proceedings of the 11th National Conference on Impact Dynamics. Xi'an: Northwest Institute of Nuclear Technology, 2013. (in Chinese)
[27]	解江, 李翰, 周书婷, 等. 爆炸冲击载荷下航空铝合金平板动态响应数值分析方法[J]. 应用数学和力学, 2017, 38(4): 410-420. doi: 10.21656/1000-0887.370252 XIE Jiang, LI Han, ZHOU Shuting, et al. A numerical method for dynamic responses of aviation aluminum alloy plates under blast loads[J]. Applied Mathematics and Mechanics, 2017, 38(4): 410-420. (in Chinese) doi: 10.21656/1000-0887.370252