C/SiC编织型复合材料热/力学性能的多尺度预测

张永正; 刘磊; 刘琦; 徐光魁

doi:10.21656/1000-0887.440056

C/SiC编织型复合材料热/力学性能的多尺度预测

doi: 10.21656/1000-0887.440056

张永正^1,,
刘磊^2, ,,
刘琦^2,,
徐光魁^1,

1.
西安交通大学航天航空学院, 西安 710049
2.
空气动力学国家重点实验室, 四川绵阳 621000

(我刊编委刘少宝推荐)

基金项目:

国家自然科学基金项目 11972359

详细信息

作者简介:
张永正(1996—)，男，硕士生(E-mail: 1303584072@qq.com)

刘琦(1989—)，男，博士(E-mail: liuqi202@hrbeu.edu.cn)

徐光魁(1986—)，男，教授(E-mail: guangkuixu@mail.xjtu.edu.cn)

通讯作者:
刘磊(1982—)，男，副研究员(通讯作者. E-mail: l.liu@cardc.cn)

中图分类号: O34
计量
- 文章访问数: 590
- HTML全文浏览量: 462
- PDF下载量: 131
- 被引次数: 0
出版历程
- 收稿日期: 2023-03-06
- 修回日期: 2023-07-14
- 刊出日期: 2023-10-31

Multi-Scale Prediction of Thermal and Mechanical Properties of C/SiC Braided Composites

ZHANG Yongzheng^1
,,
LIU Lei^{2
, ,},
LIU Qi^2
,,
XU Guangkui^1
,

1.
School of Aerospace Engineering, Xi'an Jiaotong University, Xi'an 710049, P.R.China
2.
State Key Laboratory of Aerodynamics, Mianyang, Sichuang 621000, P.R.China

(Recommended by LIU Shaobao, M. AMM Editorial Board)

摘要

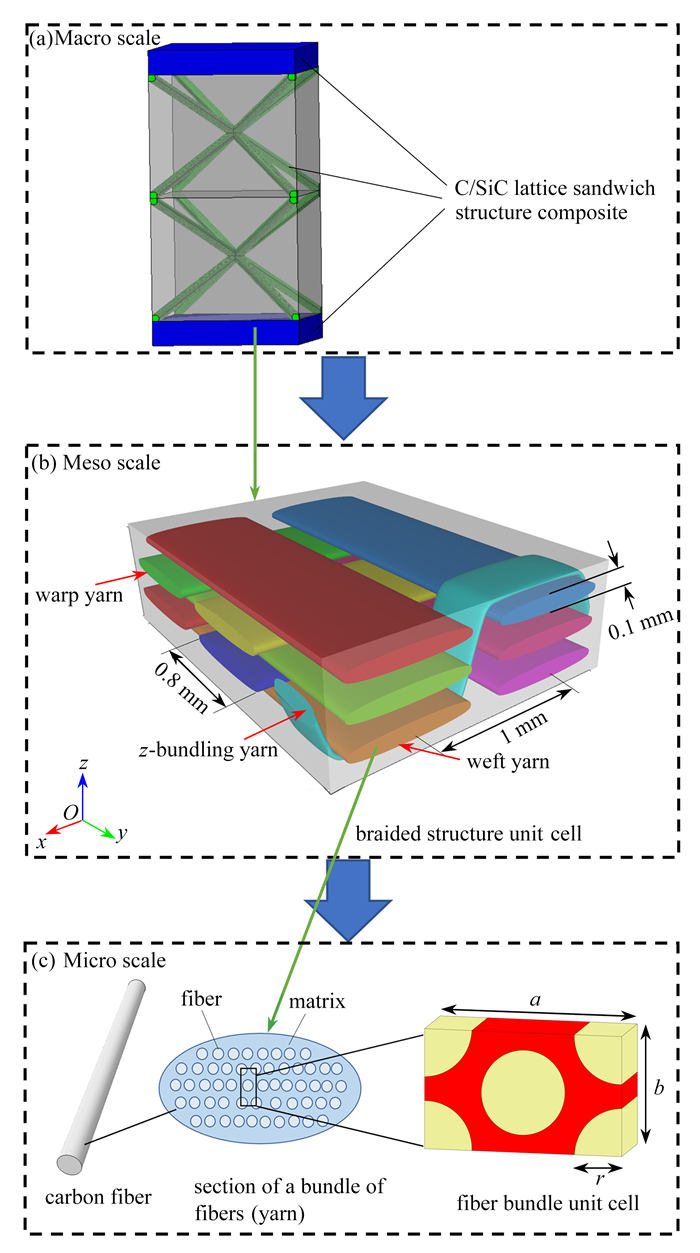
摘要: C/SiC复合材料以其优异的力学和热学性能，在航空航天、国防和化学工业等领域都得到了广泛的应用. 精准获得C/SiC复合材料的热/力学性能，对该类材料在相关领域的高效应用至关重要. 基于代表性体积单元(RVE)和周期性边界条件，考虑纤维束的体积分数、交织方式、编织维度等非均匀、多尺度特征，建立了C/SiC复合材料的微/细观单胞模型. 使用有限元软件ABAQUS在微观尺度预测了纤维束的热/力学性能，将纤维束性能引入细观模型中，分析获得了该复合材料的宏观热/力学性能. 基于此多尺度关联分析方法，在27~1 227 ℃的工作温度范围内，进一步研究了纤维束和C/SiC复合材料的热导率和热膨胀系数随温度的变化规律. 该研究对C/SiC复合材料在工程上的应用具有一定的指导意义.
- C/SiC复合材料 /
- 多尺度建模 /
- 有限元分析 /
- 周期性边界条件 /
- 热/力学性能
Abstract: C/SiC composites have been widely used in aerospace, national defense, and chemical industries due to their excellent mechanical and thermal properties. Accurate knowledge about the mechanical/thermal properties of C/SiC composites is very important for their efficient application in related fields. Based on the representative volume element (RVE) and periodic boundary conditions, a micro/meso single-cell model for C/SiC composites was established in view of the non-uniform and multi-scale characteristics of fiber bundles, such as the volume fraction, the interweaving mode, and the weaving dimension. The finite element software ABAQUS was used to predict the micro-scale thermal and mechanical properties of the fiber bundle, and the fiber bundle properties were introduced into the mesoscopic model to analyze and obtain the macroscopic thermal and mechanical properties of the composite. Based on this multi-scale correlation analysis method, the thermal conductivity and thermal expansion coefficient of fiber bundles and C/SiC composites were further studied at the operating temperatures ranging from 27~1 227 ℃. The study has certain guiding significance for the application of C/SiC composites in engineering.
- C/SiC composite /
- multi-scale modeling /
- finite element analysis /
- periodic boundary condition /
- thermal/mechanical property
edited-by

edited-by
注释:

1) (我刊编委刘少宝推荐)

HTML全文

图 1 三维C/SiC复合材料的多尺度有限元模型

Figure 1. The multi-scale finite element model for 3D C/SiC composites

下载: 全尺寸图片幻灯片

图 2 细观尺度RVE的网格

Figure 2. Meshes of the mesoscale RVE

下载: 全尺寸图片幻灯片

图 3 周期性边界条件

Figure 3. Periodic boundary conditions

下载: 全尺寸图片幻灯片

图 4 计算径向热导率时的边界条件

Figure 4. Boundary conditions for calculating radial thermal conductivity

下载: 全尺寸图片幻灯片

图 5 计算轴向热导率时的边界条件

Figure 5. Boundary conditions for calculating axial thermal conductivity

下载: 全尺寸图片幻灯片

图 6 3D正交互锁编织型复合材料(单位: mm)

Figure 6. The 3D positive interlocking braided composite(unit: mm)

下载: 全尺寸图片幻灯片

图 7 3D正交互锁编织型复合材料单胞的网格模型

Figure 7. The mesh model for 3D positive interlocking braided composite cells

下载: 全尺寸图片幻灯片

图 8 弹性模量E₁₁和Poisson比μ₁₂的数值和实验结果的比较

Figure 8. Comparison of the values of Young's modulus E₁₁ and Poisson's ratio μ₁₂ with the experimental results

下载: 全尺寸图片幻灯片

图 9 细观尺度下5种单胞模型

Figure 9. 5 mesoscale single-cell models

下载: 全尺寸图片幻灯片

图 10 2D 1×1编织结构热/力学系数随纤维束体积分数的变化

Figure 10. Variations of thermal/mechanical coefficients of 2D 1×1 braided structure with the fiber bundle volume fraction

下载: 全尺寸图片幻灯片

图 11 5种编织结构的热导率

Figure 11. Thermal conductivities of 5 braided structures

下载: 全尺寸图片幻灯片

图 12 5种编织结构的热膨胀系数

Figure 12. Thermal expansion coefficient of 5 braided structures

下载: 全尺寸图片幻灯片

表 1 单位应变边界条件

Table 1. Unit strain boundary conditions

condition	ε_x	ε_y	ε_z	γ_xy	γ_xz	γ_yz
1	1	0	0	0	0	0
2	0	1	0	0	0	0
3	0	0	1	0	0	0
4	0	0	0	1	0	0
5	0	0	0	0	1	0
6	0	0	0	0	0	1

下载: 导出CSV

表 2 碳纤维和环氧树脂的属性

Table 2. Properties of the carbon fiber and the epoxy resin

property	EP828	T-900
E₁/GPa	3.40	294
E₂/GPa	3.40	6.50
G₁₂/GPa	1.26	18.2
G₂₃/GPa	1.26	6.50
μ₁₂	0.35	0.32
μ₂₃	0.35	0.41
α₁₁/℃^-1	6.45×10^-5	-5.6×10^-7
α₂₂/℃^-1	6.45×10^-5	5.6×10^-6
α₃₃/℃^-1	6.45×10^-5	5.6×10^-6

下载: 导出CSV

表 3 纱线热/力学性能的预测结果

Table 3. Predicted results of thermal/mechanical properties of yarns

property	value (V_f=50%)
property	our method	AEH method^[21]
E₁/GPa	4.96	4.96
E₂/GPa	4.96	4.96
E₃/GPa	148.63	148.70
G₁₂/GPa	2.44	2.45
G₁₃/GPa	3.20	3.21
G₂₃/GPa	3.20	3.21
μ₁₂	0.475	0.476
μ₁₃	0.336	0.335
μ₂₃	0.011	0.011
α₁₁/℃^-1	4.5×10^-5	4.5×10^-5
α₂₂/℃^-1	4.5×10^-5	4.5×10^-5
α₃₃/℃^-1	2.0×10^-7	2.0×10^-7

下载: 导出CSV

表 4 三维正交编织复合材料热/力学性能的预测结果

Table 4. Prediction results of thermal/mechanical properties of 3D orthogonal braided composite

property	our method	FEM method^[16]
E₁/GPa	34.10	32.97
E₂/GPa	52.01	51.21
E₃/GPa	11.85	11.13
G₁₂/GPa	2.94	2.88
G₁₃/GPa	2.51	2.43
G₂₃/GPa	2.53	2.45
μ₁₂	0.062 0	0.061 7
μ₁₃	0.486 0	0.497 0
μ₂₃	0.441 0	0.456 0
α₁₁/℃^-1	1.310×10^-5	12.920×10^-5
α₂₂/℃^-1	6.930×10^-6	6.890×10^-6
α₃₃/℃^-1	8.110×10^-6	7.940×10^-6

下载: 导出CSV

表 5 纤维束的弹性常数

Table 5. Elastic constants of fiber bundles

E₁/GPa	E₂/GPa	E₃/GPa	G₁₂/GPa	G₁₃/GPa	G₂₃/GPa	μ₁₂	μ₁₃	μ₂₃
308.24	76.59	76.59	43.02	43.02	25.44	0.28	0.28	0.41

下载: 导出CSV

表 6 纤维束的热膨胀系数和热导率

Table 6. Thermal expansion coefficients and thermal conductivities of the fiber bundle

T/℃	α₁₁/℃^-1	(α₂₂=α₃₃)/℃^-1	k₁₁/(W·m^-1·℃^-1)	(k₂₂=k₃₃)/(W·m^-1·℃^-1)
27	3.80×10^-5	4.48×10^-6	147.17	83.84
227	1.00×10^-6	4.90×10^-6	142.91	79.66
427	1.72×10^-6	5.65×10^-6	138.32	72.07
627	2.06×10^-6	5.92×10^-6	133.77	64.85
827	2.36×10^-6	6.18×10^-6	131.13	60.21
1 027	2.64×10^-6	6.42×10^-6	125.42	54.52
1 227	2.90×10^-6	6.69×10^-6	118.61	45.06

下载: 导出CSV

表 7 5种编织结构的弹性常数

Table 7. Elastic constants of 5 braided structures

braided structure	E₁/GPa	E₂/GPa	E₃/GPa	G₁₂/GPa	G₁₃/GPa	G₂₃/GPa
1×1 2D	299.22	299.22	161.23	92.50	56.40	56.40
2×1 2D	299.94	299.94	161.80	92.71	56.49	56.49
2×2 2D	298.23	298.23	160.18	91.77	56.15	56.15
2.5D coss-linking	280.73	300.09	169.10	91.70	57.37	63.16
3D orthogonal	289.49	322.89	175.19	96.76	56.60	66.04

下载: 导出CSV

参考文献(26)

[1]	XU Y, REN S, ZHANG W, et al. Study of thermal buckling behavior of plain woven C/SiC composite plate using digital image correlation technique and finite element simulation[J]. Thin-Walled Structures, 2018, 131(10): 385-392.
[2]	汪海滨, 张卫红, 许英杰. C/C-SiC机织复合材料尺度参数对力学性能的影响[J]. 复合材料学报, 2010, 27(5): 93-100. https://www.cnki.com.cn/Article/CJFDTOTAL-FUHE201005017.htm WANG Haibin, ZHANG Weihong, XU Yingjie. Effects of different scale parameters on effective modulus of woven C/C-SiC composites[J]. Acta Materiae Compositae Sinica, 2010, 27(5): 93-100. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-FUHE201005017.htm
[3]	梅辉. 2D C/SiC在复杂耦合环境中的损伤演变和失效机制[D]. 硕士学位论文. 西安: 西北工业大学, 2007. MEI Hui. Damage evolution and failure mechanism of 2D C/SiC in complex coupled environment[D]. Master Thesis. Xi'an: Northwestern Polytechnical University, 2007. (in Chinese)
[4]	YANG Q, HAN X, XU C, et al. Development and validation of an anisotropic damage constitutive model for C/SiC composite[J]. Ceramics International, 2018, 44(18): 22880-22889. doi: 10.1016/j.ceramint.2018.09.081
[5]	刘波, 雷友锋, 宋迎东. 纤维增强复合材料宏观与细观统一的细观力学模型[J]. 航空发动机, 2007, 3: 45-49. https://www.cnki.com.cn/Article/CJFDTOTAL-HKFJ200703011.htm LIU Bo, LEI Youfeng, SONG Yingdong. A unified micromechanical model of macroscopical and microscopical fiber reinforced composites[J]. Aeroengine, 2007, 3: 45-49. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-HKFJ200703011.htm
[6]	XU Y, ZHANG P, LU H, et al. Hierarchically modeling the elastic properties of 2D needled carbon/carbon composites[J]. Composite Structures, 2015, 133: 148-156. doi: 10.1016/j.compstruct.2015.07.081
[7]	王新峰, 周光明, 周储伟, 等. 基于周期性边界条件的机织复合材料多尺度分析[J]. 南京航空航天大学学报, 2005, 37(6): 730-735. doi: 10.3969/j.issn.1005-2615.2005.06.012 WANG Xinfeng, ZHOU Guangming, ZHOU Chuwei, et al. Multi-scale analysis of woven composites based on periodic boundary conditions[J]. Transactions of Nanjing University of Aeronautics and Astronautics, 2005, 37(6): 730-735. (in Chinese) doi: 10.3969/j.issn.1005-2615.2005.06.012
[8]	XU Y, ZHANG P, ZHANG W. Two-scale micromechanical modeling of the time dependent relaxation modulus of plain weave polymer matrix composites[J]. Composite Structures, 2015, 123: 35-44. doi: 10.1016/j.compstruct.2014.12.031
[9]	ISHIKAWA T, LI Z S, LU Y P, et al. The GS-X pump in plant, yeast, and animal cells: structure, function, and gene expression[J]. Bioscience Reports, 1997, 17(2): 189-207. doi: 10.1023/A:1027385513483
[10]	崔春丽, 徐耀玲. 预测纳米纤维复合材料有效弹性性能的界面模型和界面相模型[J]. 应用数学和力学, 2022, 43(8): 877-887. doi: 10.21656/1000-0887.420231 CUI Chunli, XU Yaoling. Interfacial model and interfacial phase model for predicting effective elastic properties of nanofiber composites[J]. Applied Mathematics and Mechanics, 2022, 43(8): 877-887. (in Chinese) doi: 10.21656/1000-0887.420231
[11]	李典森, 卢子兴, 卢文书. 三维四向编织复合材料刚度和强度的理论预测[J]. 应用数学和力学, 2008, 29(2): 149-156. http://www.applmathmech.cn/article/id/1030 LI Diansen, LU Zixing, LU Wenshu. Theoretical prediction of stiffness and strength of three-dimensional four-way braided composites[J]. Applied Mathematics and Mechanics, 2008, 29(2): 149-156. (in Chinese) http://www.applmathmech.cn/article/id/1030
[12]	张春春, 王艳超, 黄争鸣. 横观各向同性基体复合材料的等效弹性常数[J]. 应用数学和力学, 2018, 39(7): 750-765. doi: 10.21656/1000-0887.380267 ZHANG Chunchun, WANG Yanchao, HUANG Zhengming. Equivalent elastic constants of transverse isotropic matrix composites[J]. Applied Mathematics and Mechanics, 2018, 39(7): 750-765. (in Chinese) doi: 10.21656/1000-0887.380267
[13]	NAIK N K, SRIDEVI E. An analytical method for thermoelastic analysis of 3D orthogonal interlock woven composites[J]. Journal of Reinforced Plastics and Composites, 2002, 21(13): 1149-1191. doi: 10.1177/073168402128987716
[14]	AI S, FU H, HE R, et al. Multi-scale modeling of thermal expansion coefficients of C/C composites at high temperature[J]. Materials and Design, 2015, 82(5): 181-188.
[15]	ULLAH Z, ZHOU X Y, KACZMARCZYK L, et al. A unified framework for the multi-scale computational homogenisation 3D-textile composites[J]. Composites (Part B): Engineering, 2019, 167: 582-598. doi: 10.1016/j.compositesb.2019.03.027
[16]	SIDDGONDE N, GHOSH A. Thermo-mechanical modeling of C/C 3D orthogonal and angle interlock woven fabric composites in high temperature environment[J]. Mechanics of Materials, 2020, 148: 1-14.
[17]	赵玉芬. 三维机织复合材料热传导及力学性能的多尺度有限元分析[D]. 硕士学位论文. 天津: 天津工业大学, 2017. ZHAO Yufen. Multi-scale finite element analysis of heat conduction and mechanical properties of three-dimensional woven composites[D]. Master Thesis. Tianjin: Tianjin Polytechnic University, 2017. (in Chinese)
[18]	张超, 许希武, 严雪. 纺织复合材料细观力学分析的一般性周期性边界条件及其有限元实现[J]. 航空学报, 2013, 34(7): 1636-1645. https://www.cnki.com.cn/Article/CJFDTOTAL-HKXB201307016.htm ZHANG Chao, XU Xiwu, YAN Xue. General periodic boundary conditions and finite element realization of textile composites for meso-mechanical analysis[J]. Acta Aeronautica et Astronautica Sinica, 2013, 34(7): 1636-1645. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-HKXB201307016.htm
[19]	徐焜, 许希武. 三维编织复合材料弹性性能数值预测及细观应力分析[J]. 复合材料学报, 2007, 24(3): 178-185. https://www.cnki.com.cn/Article/CJFDTOTAL-FUHE200703032.htm XU Kun, XU Xiwu. Prediction of elastic constants and simulation of stress field of 3D braided composites based on the finite element method[J]. Acta Materiae Compositae Sinica, 2007, 24(3): 178-185. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-FUHE200703032.htm
[20]	LIU Y, QU Z G, GUO J, et al. Numerical study on effective thermal conductivities of plain woven C/SiC composites with considering pores in interlaced woven yarns[J]. International Journal of Heat and Mass Transfer, 2019, 140: 410-419.
[21]	MREN A, NW A, AKA B, et al. Thermomechanical properties and stress analysis of 3-D textile composites by asymptotic expansion homogenization method[J]. Composites (Part B): Engineering, 2014, 60: 378-391.
[22]	PRADÈRE C, BATSALE J C, GOYHENECHE J M, et al. Thermal properties of carbon fibers at very high temperature[J]. Carbon, 2009, 47(3): 737-743.
[23]	PRADERE C, SAUDER C. Transverse and longitudinal coefficient of thermal expansion of carbon fibers at high temperatures (300~2 500 K)[J]. Carbon, 2008, 46(14): 1874-1884.
[24]	SAUDER C, LAMON J, SAUDER C, et al. Thermomechanical properties of carbon fibres at high temperatures (up to 2 000 ℃)[J]. Composites Science and Technology, 2002, 62(4): 499-504.
[25]	BOWLES D, TOMPKINS S S. Prediction of coefficients of thermal expansion for unidirectional composites[J]. Journal of Composite Materials, 1989, 23: 370-388.
[26]	BORKOWSKI L, CHATTOPADHYAY A. Multiscale model of woven ceramic matrix composites considering manufacturing induced damage[J]. Composite Structures, 2015, 126: 62-71.

施引文献

资源附件(0)

访问统计

图(12) / 表(7)

计量

文章访问数: 590
HTML全文浏览量: 462
PDF下载量: 131
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

留言板

C/SiC编织型复合材料热/力学性能的多尺度预测

doi: 10.21656/1000-0887.440056

作者简介:
张永正(1996—)，男，硕士生(E-mail: 1303584072@qq.com)

刘琦(1989—)，男，博士(E-mail: liuqi202@hrbeu.edu.cn)

徐光魁(1986—)，男，教授(E-mail: guangkuixu@mail.xjtu.edu.cn)

通讯作者:
刘磊(1982—)，男，副研究员(通讯作者. E-mail: l.liu@cardc.cn)

计量

Multi-Scale Prediction of Thermal and Mechanical Properties of C/SiC Braided Composites

计量

目录

留言板

C/SiC编织型复合材料热/力学性能的多尺度预测

doi: 10.21656/1000-0887.440056

作者简介: 张永正(1996—)，男，硕士生(E-mail: 1303584072@qq.com) 刘琦(1989—)，男，博士(E-mail: liuqi202@hrbeu.edu.cn) 徐光魁(1986—)，男，教授(E-mail: guangkuixu@mail.xjtu.edu.cn)

通讯作者: 刘磊(1982—)，男，副研究员(通讯作者. E-mail: l.liu@cardc.cn)

计量

出版历程

Multi-Scale Prediction of Thermal and Mechanical Properties of C/SiC Braided Composites

计量

出版历程

目录

作者简介:
张永正(1996—)，男，硕士生(E-mail: 1303584072@qq.com)

刘琦(1989—)，男，博士(E-mail: liuqi202@hrbeu.edu.cn)

徐光魁(1986—)，男，教授(E-mail: guangkuixu@mail.xjtu.edu.cn)

通讯作者:
刘磊(1982—)，男，副研究员(通讯作者. E-mail: l.liu@cardc.cn)