An Iterative Regularization Method for Solving Backward Problems With 2 Perturbation Data
-
摘要: 该文考虑了一类带有扰动扩散系数和扰动终值数据的空间分数阶扩散方程反向问题，从终值时刻的测量数据来反演初始时刻数据. 该问题是严重不适定的，因此该文提出了一种迭代正则化方法来处理该反向问题，并利用先验正则化参数选取规则得到了正则化解和精确解之间的误差估计，最后进行了一些数值模拟，验证了方法的有效性.Abstract: The backward problem of space-fractional diffusion equations with perturbed diffusion coefficients and perturbed final data was considered. The initial data were recovered from the measured data at the final time. Given the severe ill-posedness of this problem, an iterative regularization method was proposed to tackle it. The convergence error estimate between the exact and approximate solutions was obtained under the assumption of an a-priori bound on the exact solution. Finally, several numerical simulations were conducted to verify the effectiveness of this method.
-
图 1 精确解和在不同误差水平下的正则化解
Figure 1. Exact solutions and regularized solutions with different noise levels
图 2 不同时刻下的精确解和正则化解
Figure 2. Comparison of exact solutions and regularized solutions at different moments
表 1 不同误差水平下u(x, t)的相对误差和绝对误差(α=0.6)
Table 1. Relative and absolute errors corresponding to different noise levels(α=0.6)
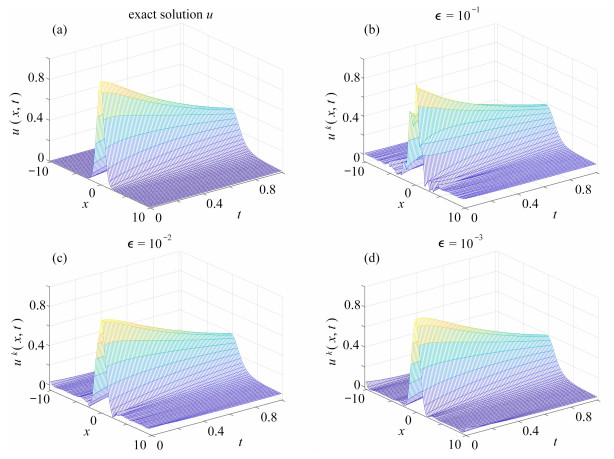
t ε1=10-1 ε2=0 ε1=0 ε2=10-1 ε1=10-1 ε2=10-1 ea(t) er(t) ea(t) er(t) ea(t) er(t) 0.9 0.017 0 0.032 5 0.023 8 0.045 4 0.024 8 0.047 4 0.5 0.076 9 0.062 8 0.074 3 0.068 8 0.093 5 0.076 4 0.3 0.114 0 0.087 3 0.109 8 0.084 6 0.137 3 0.096 0 t ε1=10-2 ε2=0 ε1=0 ε2=10-2 ε1=10-2 ε2=10-2 ea(t) er(t) ea(t) er(t) ea(t) er(t) 0.9 0.007 5 0.014 3 0.007 3 0.014 0 0.007 8 0.015 0 0.5 0.041 6 0.034 0 0.035 5 0.029 0 0.044 8 0.036 6 0.3 0.078 9 0.051 4 0.068 7 0.044 7 0.083 1 0.054 2 
下载: 导出CSV
表 2 t=0.9时刻下β, ε与迭代步数k之间的关系
Table 2. Relationships between β, ε and iterative step number k at time t=0.9
β=0.1 β=0.5 β=1 k(ε=10-1) 68 15 7 k(ε=10-2) 418 126 18 k(ε=10-3) 549 193 56
下载: 导出CSV
表 3 Tikhonov正则化方法(TRM)和迭代正则化方法(IRM)下u(x, t)的相对误差和绝对误差(α=0.6)
Table 3. Relative and absolute errors of u(x, t) under the TRM and IRM(α=0.6)
t=0.8 t=0.5 t=0.3 ε2=10-1 ε1=10-2 ε2=10-1 ε1=10-2 ε2=10-1 ε1=10-2 TRM ea(t) 0.079 6 0.018 5 0.097 2 0.028 8 0.260 9 0.048 0 er(t) 0.108 4 0.025 1 0.115 8 0.027 3 0.168 9 0.031 0 IRM ea(t) 0.033 0 0.003 4 0.060 9 0.011 6 0.137 3 0.060 4 er(t) 0.045 3 0.004 6 0.049 7 0.009 1 0.096 0 0.035 7
下载: 导出CSV
-
[1] METZLER R, KLAFTER J. The restaurant at the end of the random walk: recent developments in the description of anomalous transport by fractional dynamics[J]. Journal of Physics A: Mathematical and General, 2004, 3737 (87): 161-208. [2] HALL M G, BARRICK T R. From diffusion-weighted MRI to anomalous diffusion imaging[J]. Magnetic Resonance in Medicine, 2008, 59 (3): 447-455. doi: 10.1002/mrm.21453 [3] BENSON D A, WHEATCRAFT S W, MEERSCHAERT M M. Application of a fractional advection-dispersion equation[J]. Water Resources Research, 2000, 36 (6): 1403-1412. doi: 10.1029/2000WR900031 [4] 余钊圣, 林建忠. 粘弹性二阶流体混合层流场拟序结构的数值研究[J]. 应用数学和力学, 1998, 19 (8): 671-677. http://www.applmathmech.cn/article/id/2445YU Zhaosheng, LIN Jianzhong. Numerical research on the coherent structure in the viscoelastic second-order mixing layers[J]. Applied Mathematics and Mechanics, 1998, 19 (8): 671-677. (in Chinese) http://www.applmathmech.cn/article/id/2445 [5] KOENDERINK J J. The structure of images[J]. Biological Cybernetics, 1984, 50 (5): 363-370. doi: 10.1007/BF00336961 [6] ATMADJA J, BAGTZOGLOU A C. Pollution source identification in heterogeneous porous media[J]. Water Resources Research, 2001, 37 (8): 2113-2125. doi: 10.1029/2001WR000223 [7] FENG X L, ZHAO M X, QIAN Z. A Tikhonov regularization method for solving a backward time-space fractional diffusion problem[J]. Journal of Computational and Applied Mathematics, 2022, 411 : 114236. doi: 10.1016/j.cam.2022.114236 [8] KHIEU T T, VO H H. Recovering the historical distribution for nonlinear space-fractional diffusion equation with temporally dependent thermal conductivity in higher dimensional space[J]. Journal of Computational and Applied Mathematics, 2019, 345 : 114-126. doi: 10.1016/j.cam.2018.06.018 [9] YANG F, PU Q, LI X X. The fractional Landweber method for identifying the space source term problem for time-space fractional diffusion equation[J]. Numerical Algorithms, 2021, 87 (3): 1229-1255. doi: 10.1007/s11075-020-01006-4 [10] YANG F, WANG N, LI X X. A quasi-boundary regularization method for identifying the initial value of time-fractional diffusion equation on spherically symmetric domain[J]. Journal of Inverse and Ill-Posed Problems, 2019, 27 (5): 609-621. doi: 10.1515/jiip-2018-0050 [11] 赵丽志, 冯晓莉. 一类随机对流扩散方程的反源问题[J]. 应用数学和力学, 2022, 43 (12): 1392-1401. doi: 10.21656/1000-0887.420399ZHAO Lizhi, FENG Xiaoli. The inverse source problem for a class of stochastic convection-diffusion equations[J]. Applied Mathematics and Mechanics, 2022, 43 (12): 1392-1401. (in Chinese) doi: 10.21656/1000-0887.420399 [12] SHI C, WANG C, ZHENG G, et al. A new a posteriori parameter choice strategy for the convolution regularization of the space-fractional backward diffusion problem[J]. Journal of Computational and Applied Mathematics, 2015, 279 : 233-248. doi: 10.1016/j.cam.2014.11.013 [13] TUAN N H, KIRANE M, BIN M B, et al. Filter regularization for final value fractional diffusion problem with deterministic and random noise[J]. Computers and Mathematics With Applications, 2017, 74 (6): 1340-1361. doi: 10.1016/j.camwa.2017.06.014 [14] 柳冕, 程浩, 石成鑫. 一类非线性时间分数阶扩散方程反问题的变分型正则化[J]. 应用数学和力学, 2022, 43 (3): 341-352. doi: 10.21656/1000-0887.420168LIU Mian, CHENG Hao, SHI Chengxin. Variational regularization of the inverse problem of a class of nonlinear time-fractional diffusion equations[J]. Applied Mathematics and Mechanics, 2022, 43 (3): 341-352. (in Chinese) doi: 10.21656/1000-0887.420168 [15] NEZZA E D, PALATUCCI G, VALDINOCI E. Hitchhiker's guide to the fractional Sobolev spaces[J]. Bulletin Des Sciences Mathematiques, 2012, 136 (5): 521-573. doi: 10.1016/j.bulsci.2011.12.004 [16] ZHENG G H, WEI T. Two regularization methods for solving a Riesz-Feller space-fractional backward diffusion problem[J]. Inverse Problems, 2010, 26 (11): 115017. doi: 10.1088/0266-5611/26/11/115017 [17] ZHENG G H, ZHANG Q G. Determining the initial distribution in space-fractional diffusion by a negative exponential regularization method[J]. Inverse Problems in Science and Engineering, 2017, 25 (7): 965-977. doi: 10.1080/17415977.2016.1209750 [18] ZHAO J, LIU S, LIU T. An inverse problem for space-fractional backward diffusion problem[J]. Mathematical Methods in the Applied Sciences, 2014, 37 (8): 1147-1158. doi: 10.1002/mma.2876 [19] LUAN T N, KHANH T Q. Determination of initial distribution for a space-fractional diffusion equation with time-dependent diffusivity[J]. Bulletin of the Malaysian Mathematical Sciences Society, 2021, 44 (5): 3461-3487. doi: 10.1007/s40840-021-01118-7 [20] TUAN N H, HAI D N D, KIRANE M. On a Riesz-Feller space fractional backward diffusion problem with a nonlinear source[J]. Journal of Computational and Applied Mathematics, 2017, 312 : 103-126. doi: 10.1016/j.cam.2016.01.003 [21] TUAN N H, TRONG D D, HAI D N D, et al. A Riesz-Feller space-fractional backward diffusion problem with a time-dependent coefficient: regularization and error estimates[J]. Mathematical Methods in the Applied Sciences, 2017, 40 (11): 4040-4064. doi: 10.1002/mma.4284 [22] DIEN N M, TRONG D D. The backward problem for nonlinear fractional diffusion equation with time-dependent order[J]. Bulletin of the Malaysian Mathematical Sciences Society, 2021, 44 (5): 3345-3359. doi: 10.1007/s40840-021-01113-y [23] CHENG H, FU C L. An iteration regularization for a time-fractional inverse diffusion problem[J]. Applied Mathematical Modelling, 2012, 36 (11): 5642-5649. doi: 10.1016/j.apm.2012.01.016 [24] DENG Y, LIU Z. Iteration methods on sideways parabolic equations[J]. Inverse Problems, 2009, 25 (9): 095004. doi: 10.1088/0266-5611/25/9/095004 [25] DENG Y, LIU Z. New fast iteration for determining surface temperature and heat flux of general sideways parabolic equation[J]. Nonlinear Analysis: Real World Applications, 2011, 12 (1): 156-166. doi: 10.1016/j.nonrwa.2010.06.005 [26] WANG J G, WEI T. An iterative method for backward time-fractional diffusion problem[J]. Numerical Methods for Partial Differential Equations, 2014, 30 (6): 2029-2041. doi: 10.1002/num.21887 [27] 孙志忠, 高广花. 分数阶微分方程的有限差分法[M]. 北京: 科学出版社, 2015.SUN Zhizhong, GAO Guanghua. Finite Difference Method for Fractional Differential Equations[M]. Beijing: Science Press, 2015. (in Chinese) [28] SAICHEV A I, ZASLAVAKY G M. Fractional kinetic equations: solutions and applications[J]. Chaos, 1997, 7 (4): 753-764. doi: 10.1063/1.166272 [29] ÇELIK C, DUMAN M. Crank-Nicolson method for the fractional diffusion equation with the Riesz fractional derivative[J]. Journal of Computational Physics, 2012, 231 (4): 1743-1750. doi: 10.1016/j.jcp.2011.11.008 -
图(2) / 表(3)
计量
- 文章访问数: 452
- HTML全文浏览量: 146
- PDF下载量: 59
- 被引次数: 0
渝公网安备50010802005915号