Research on the Dynamic Behaviors of the Vortex Induced Vibration Power Generation System Under Nonlinear Restoring Forces
-
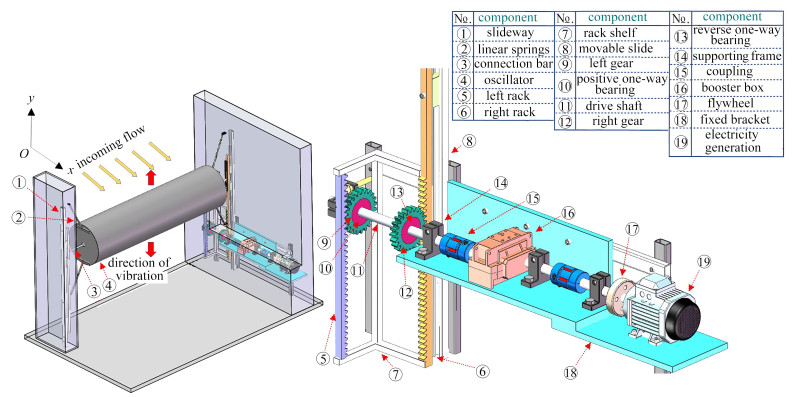
摘要: 利用线性弹簧斜向布置的几何非线性产生非线性恢复力,提出了引入非线性恢复力的水下涡激振动(VIV)发电系统. 该系统通过单向轴承、齿轮齿条机构、增速箱和转子发电机,将钝体横向往复运动转变为发电机的单向旋转运动. 建立了综合考虑流-固-电耦合的水下涡激振动发电系统动力学方程,利用非线性振动理论,获得了钝体非线性振动的静态平衡点分岔和不同稳态运动的区间,重点研究了PF-2SN和2PF-2SN两种静态分岔情况下钝体的非线性动力学行为,获得了不同流速下钝体振动的Poincaré映射、相图和幅频图,分析了钝体在单周期小幅运动、大幅混沌运动和准周期大幅运动等运动模式下的振动行为及运动规律,并计算了在钝体处于不同稳态运动时的发电机功率. 结果表明:在PF-2SN分岔方式中,系统处于二稳态运动时的振动和发电具有明显优势,平均振幅比为2.18、发电功率最大值为24.45 W. 而在2PF-2SN分岔方式中,系统处于三稳态运动时的振动和发电更具优势,平均振幅比为1.98、发电功率最大值为18.32 W.Abstract: An underwater vortex-induced vibration power generation system under nonlinear restoring forces was proposed. The nonlinear restoring force was generated by means of the geometrical nonlinearity of linear springs arranged obliquely. The lateral reciprocating motion of the oscillator was transformed into a unidirectional rotary motion of the generator by dint of unidirectional bearings and gear-rack mechanisms, a booster box and a rotor generator. The dynamic flow-structure-electricity coupling equations for the vortex-induced vibration power generation system were established. Then the static equilibrium point bifurcation of the nonlinear vibration of the oscillator and the ranges of different stable state motions were obtained under the nonlinear vibration theory. The nonlinear dynamic behaviors of the oscillator under the conditions of PF-2SN and 2PF-2SN bifurcations were studied mainly. The bifurcation graphs, phase graphs and Poincaré mappings of the system were achieved. The vibration behaviors and motion laws of the oscillator under the conditions of single-period small motion, large chaos motion and quasi-periodic large motion were analyzed. Then, the generation power values of the generator for different stable state motions of the oscillator were also calculated. The results show that, in the PF-2SN bifurcation mode, the system has obvious advantages in vibration and power generation in the bi-stable motion, with an average amplitude ratio of 2.18 and a maximum power of 24.45 W. While in the 2PF-2SN bifurcation mode, the vibration and power generation of the system are more superior in the tri-stable motion, with an average amplitude ratio of 1.98 and a maximum power generation of 18.32 W.
-
表 1 系统结构及物理参数表
Table 1. Structural and physical parameters of the system
parameter symbol value parameter symbol value oscillator mass ms/kg 50 spring length Ls/m 0.4 structural damping cs/(N·s·m-1) 20 seawater density ρ/(kg·m-3) 1 040 additional quality factor Cm[21] 1 oscillator length L/m 1.6 viscous force coefficient γ[21] 0.8 oscillator diameter D/m 0.3 fluid-structure coupling parameter A[20] 12 Strouhal number Sr[21] 0.2 (left/right) gear radius r/m 0.05 total moment of inertia J/(kg·m2) 0.625 booster ratio nb 3.3 Van der Pol parameter ε[21] 0.3 generator internal resistance Ra/Ω 0.25 flywheel moment of inertia If/(kg·m2) 0.04 generator external resistance RL/Ω 20 generator moment of inertia Ig/(kg·m2) 0.01 voltage constant Kg/(V·s·rad-1) 0.26 torque coefficient Kt/(N·m·A-1) 0.45 equivalent damping coefficient Cs[22] 0.057 2 static cylinder lift amplitude CL0[23] 0.3 表 2 钝体运动状态汇总(a=0.1 m,b=0.1 m)
Table 2. Summary of the motion of the oscillator(a=0.1 m, b=0.1 m)
flow speed motion of the oscillator cross potential wells (yes or no) u<0.6 m/s small single-cycle motion in the potential well no 0.6 m/s≤u<0.99 m/s large chaotic motion between potential wells yes 0.99 m/s≤u<1.56 m/s large 3-cycle motion between potential wells yes 1.56 m/s≤u<1.77 m/s large quasiperiodic and chaotic motion between potential wells yes 1.77 m/s≤u<1.92 m/s small 2-cycle motion in the potential well no u≥1.92 m/s small single-cycle motion in the potential well no 表 3 钝体运动模式汇总(a=0.1 m,b=0.2 m)
Table 3. Summary of motion modes of the oscillator(a=0.1 m, b=0.2 m)
flow speed motion of the oscillator cross potential wells (yes or no) u<0.36 m/s small single-cycle motion in the potential well no 0.36≤u<0.85 m/s large chaotic motion between potential wells yes 0.85≤u<1.01 m/s 3-cycle large-scale periodic motion between potential wells yes 1.01≤u<1.12 m/s large quasi-periodic motion between potential wells yes 1.12≤u<1.34 m/s small 2-cycle motion in the potential well no u≥1.34 m/s small single-cycle motion in the potential well no 表 4 钝体运动模式汇总(a=0.1 m,b=0.15 m)
Table 4. Summary of motion modes of the oscillator (a=0.1 m, b=0.15 m)
flow speed motion of the oscillator cross potential wells (yes or no) u<0.48 m/s small single-cycle motion in the potential well no 0.48 m/s≤u<0.93 m/s large chaotic motion between potential wells yes 0.93 m/s≤u<1.13 m/s quasi 3-cycle large-scale motion between potential wells yes 1.13 m/s≤u<1.15 m/s large 6-cycle motion between potential wells yes 1.15 m/s≤u<1.26 m/s large 3-cycle motion between potential wells yes 1.26 m/s≤u<1.46 m/s large quasiperiodic and chaotic motion between potential wells yes 1.46 m/s≤u<1.61 m/s small 2-cycle motion in the potential well no u≥1.61 m/s small single-cycle motion in the potential well no 表 5 钝体运动模式汇总(a=0.2 m,b=0.15 m)
Table 5. Summary of motion modes of the oscillator (a=0.2 m, b=0.15 m)
flow speed motion of the oscillator cross potential wells (yes or no) u<0.28 m/s small single-cycle motion in the potential well no 0.28 m/s≤u<0.72 m/s large single-cycle motion between potential wells yes 0.72 m/s≤u<0.92 m/s large quasi-periodic motion between potential wells yes 0.92 m/s≤u<0.96 m/s large 5-cycle motion between potential wells yes 0.96 m/s≤u<1.01 m/s large quasi-periodic motion between potential wells yes 1.01 m/s≤u<1.03 m/s small quasi-periodic motion in the potential well no 1.03 m/s≤u<1.11 m/s small 2-cycle motion in the potential well no u≥1.11 m/s small single-cycle motion in the potential well no -
[1] 孙云卿, 吴志强, 章国齐, 等. 海洋立管双模态动力学分岔分析[J]. 应用数学和力学, 2020, 41 (5): 480-490. doi: 10.21656/1000-0887.400257 SUN Yunqing, WU Zhiqiang, ZHANG Guoqi, et al. Bifurcation analysis of dual-mode dynamics for marine risers[J]. Applied Mathematics and Mechanics, 2020, 41 (5): 480-490. (in Chinese) doi: 10.21656/1000-0887.400257 [2] 严浩, 代胡亮, 王琳, 等. 气-液横向流动下悬臂柱体结构涡激振动机理研究[J]. 应用数学和力学, 2022, 43 (5): 577-585.YAN Hao, DAI Huliang, WANG Lin, et al. A study on the vortex-induced vibration mechanism of cantilever cylinders under gas-liquid cross flows[J]. Applied Mathematics and Mechanics, 2022, 43 (5): 577-585. (in Chinese) [3] 陈艳波, 刘志慧, 吴适存, 等. 高速公路绿色能源系统体系架构初探[J]. 新型电力系统, 2024(1): 94-114.CHEN Yanbo, LIU Zhihui, WU Shicun, et al. Preliminary study on highway green energy system architecture[J]. New Type Power Systems, 2024(1): 94-114. (in Chinese) [4] 黄加勉, 蔡学志. 双碳背景下新能源在新型电力系统中的实践运用[J]. 电工技术, 2023(S1): 111-113.HUANG Jiamian, CAl Xuezhi. Practical application of new energy in new power systems under the dual carbon background[J]. Electric Engineering, 2023(S1): 111-113. (in Chinese) [5] 王丽华. "双碳" 背景下煤炭资源地区转型路径研究: 基于一体化绿色能源综合基地构建[J]. 节能与环保, 2023(10): 8-13. doi: 10.3969/j.issn.1009-539X.2023.10.002WANG Lihua. Research on the transformation path of coal resource regions under the background of "dual carbon": based on the construction of an integrated green energy comprehensive base[J]. Energy Conservation & Environmental Protection, 2023(10): 8-13. (in Chinese) doi: 10.3969/j.issn.1009-539X.2023.10.002 [6] 岳玉帅. 单约束圆柱海流能发电流致振动数值模拟研究[D]. 镇江: 江苏科技大学, 2019.YUE Yushuai. Numerical simulation of current-induced vibration of a single confined cylinder generated by ocean current energy[D]. Zhenjiang: Jiangsu University of Science and Technology, 2019. (in Chinese) [7] 李俊. 涡激振动驱动的三维圆柱水动能俘获及阵列布置优化研究[D]. 昆明: 昆明理工大学, 2023.LI Jun. Research on three-dimensional cylindrical water kinetic energy capture and array layout optimization driven by vortex induced vibration[D]. Kunming: Kunming University of Science and Technology, 2023. (in Chinese) [8] 白旭, 乐智斌, 张焱飞. 质量比对圆柱体流致振动能量捕获效率的影响[J]. 太阳能学报, 2018, 39 (12): 3325-3330.BAI Xu, LE Zhibin, ZHANG Yanfei. Effect of mass ratio on energy capture efficiency of vibration induced by cylinder flow[J]. Acta Energiae Solaris Sinica, 2018, 39 (12): 3325-3330. (in Chinese) [9] 陈芝贇. 基于被动控制与流致振动不稳定性储能装置效率的参数化研究[D]. 哈尔滨: 哈尔滨工程大学, 2019.CHEN Zhiyun. Parameterization research on the harnessed energy of nonlinear oscillators with passive controls in fluid induced motion[D]. Harbin: Harbin Engineering University, 2019. (in Chinese) [10] 及春宁, 孔令臣, 徐晓黎, 等. 附加旋转圆柱涡激振动发电装置能量获取性能研究[J]. 港工技术, 2022, 59 (6): 38-44.JI Chunning, KONG Lingchen, XU Xiaoli, et al. Study on energy harvest performance of vortex-induced vibration power generator with additional spinning cylinders[J]. Port Engineering Technology, 2022, 59 (6): 38-44. (in Chinese) [11] SHAN X, SUI G, TIAN H, et al. Numerical analysis and experiments of an underwater magnetic nonlinear energy harvester based on vortex-induced vibration[J]. Energy, 2022, 241 : 122933. doi: 10.1016/j.energy.2021.122933 [12] ZHANG W, LI X, HE Z. Two-degrees of freedom flow-induced vibration of circular cylinder with nonlinear stiffness[J]. Ocean Engineering, 2023, 286 : 115506. doi: 10.1016/j.oceaneng.2023.115506 [13] LIU J, BAO B, CHEN J, et al. Marine energy harvesting from tidal currents and offshore winds: a 2-DOF system based on flow-induced vibrations[J]. Nano Energy, 2023, 114 : 108664. doi: 10.1016/j.nanoen.2023.108664 [14] FANG S, DU H, YAN T, et al. Theoretical and experimental investigation on the advantages of auxetic nonlinear vortex-induced vibration energy harvesting[J]. Applied Energy, 2024, 356 : 122395. doi: 10.1016/j.apenergy.2023.122395 [15] BIBO A, ALHADIDI A H, DAQAQ M F. Exploiting a nonlinear restoring force to improve the performance of flow energy harvesters[J]. Journal of Applied Physics, 2015, 117 (4): 045103. doi: 10.1063/1.4906463 [16] HUYNH B H, TJAHJOWIDODO T, ZHONG Z W, et al. Numerical and experimental investigation of nonlinear vortex induced vibration energy converters[J]. Journal of Mechanical Science and Technology, 2017, 31 (8): 3715-3726. doi: 10.1007/s12206-017-0714-z [17] 高鸣源, 李守太, 孙玉华, 等. 多稳态电磁俘能系统的非线性动力学实验研究[J]. 振动工程学报, 2021, 34 (4): 775-781.GAO Mingyuan, LI Shoutai, SUN Yuhua, et al. Experimental study of non-linear dynamics of multi-stable electromagnetic energy harvesting system[J]. Journal of Vibration Engineering, 2021, 34 (4): 775-781. (in Chinese) [18] ZHANG B, SONG B, MAO Z, et al. Hydrokinetic energy harnessing by spring-mounted oscillators in FIM with different cross sections: from triangle to circle[J]. Energy, 2019, 189 : 116249. doi: 10.1016/j.energy.2019.116249 [19] FACCHINETTI M L, DE LANGRE E, BIOLLEY F. Vortex shedding modeling using diffusiveVan der Pol oscillators[J]. Comptes Rendus Mécanique, 2002, 330 (7): 451-456. doi: 10.1016/S1631-0721(02)01492-4 [20] 郑仲钦, 陈伟民. 结构与尾流非线性耦合涡激振动预测模型[J]. 海洋工程, 2012, 30 (4): 37-41.ZHENG Zhongqin, CHEN Weimin. Prediction of vortex-induced vibration of cylinder based on the nonlinear coupling of structure and wake oscillator[J]. The Ocean Engineering, 2012, 30 (4): 37-41. (in Chinese) [21] 吴子英, 常宇琛, 赵伟, 等. 三稳态电磁式涡激振动俘能装置发电性能研究[J]. 振动与冲击, 2022, 41 (13): 26-33.WU Ziying, CHANG Yuchen, ZHAO Wei, et al. Power generation performance of tri-stable state electromagnetic VIV energy harvester[J]. Journal of Vibration and Shock, 2022, 41 (13): 26-33. (in Chinese) [22] WANG L, TODARIA P, PANDEY A, et al. An electromagnetic speed bump energy harvester and its interactions with vehicles[J]. IEEE/ASME Transactions on Mechatronics, 2016, 21 (4): 1985-1994. http://ieeexplore.ieee.org/stamp/stamp.jsp?tp=&arnumber=7439847 [23] 康庄, 张橙, 付森, 等. 圆柱体涡激振动的高阶非线性振子模型研究[J]. 振动与冲击, 2018, 37 (18): 48-58.KANG Zhuang, ZHANG Cheng, FU Sen, et al. Nonlinear oscillator model for the vortex-induced vibration of a cylinder[J]. Journal of Vibration and Shock, 2018, 37 (18): 48-58. (in Chinese) [24] 张静宇. 弹性边界双稳态振子非线性动力学及控制研究[D]. 武汉: 华中科技大学, 2021.ZHANG Jingyu. Research on nonlinear dynamics and control of a bistable oscillator with elastic boundary[D]. Wuhan: Huazhong University of Science and Technology, 2021. (in Chinese) -





 下载:
下载:















 渝公网安备50010802005915号
渝公网安备50010802005915号